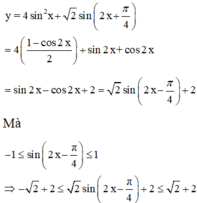Cho tan x = 5 . Tính D = 2sin2x - cos2x / 1+4sin2x

Những câu hỏi liên quan
tính giá trị biểu thức P bằng sin2-sinx*cosx+2cos2x/2sin2x-cos2x biết tan x bằng 3
Lần sau bạn vào cái hình E để gửi câu hỏi nha!
\(P=\dfrac{sin^2\alpha-sin\alpha\cdot cos\alpha+2cos^2\alpha}{2sin^2\alpha-cos^2\alpha}\)
\(P=\dfrac{tan^2\alpha-tan\alpha+2}{2tan^2\alpha-1}\) (Chia cả tử và mẫu cho \(cos^2\alpha\))
\(P=\dfrac{3^2-3+2}{2\cdot3^2-1}=\dfrac{8}{17}\)
Chúc bn học tốt!
Đúng 1
Bình luận (0)
a/ 1-sin9x+\(\sqrt{3}\)cos9x=0
b/ \(\sqrt{3}\)sin2x-2sin2x=\(\sqrt{2}\)-1
c/ sin5x+\(\sqrt{3}\)cos5x=2sin7x
d/ cos2x+sinx=\(\sqrt{3}\)(cosx-sin2x)
e/ sin2x+4sin2x+3cos2x+2=0
f/ 2sin2x+cos2x=7sinx-2cosx+4
1. sin^8(x) - cos^8(x) - 4sin^6(x) + 6sin^4(x) - 4sin^2(x) = 1
2. sin6x+sin4x+sin2x/1+cos2x+cos4x = 2sin2x
3. 1+sin2x /cosx+sinx - 1-tan^2(x/2)/1+tan^2(x/2) = sinx
4. cos4x + 4cos2x + 3 = 8cos^4(x)
5. 1+cosx+cos2x+cos3x/ 2cos^2(x)+cosx-1 = 2cosx
1. sin^8(x) - cos^8(x) - 4sin^6(x) + 6sin^4(x) - 4sin^2(x) = 1
2. sin6x+sin4x+sin2x/1+cos2x+cos4x = 2sin2x
3. 1+sin2x /cosx+sinx - 1-tan^2(x/2)/1+tan^2(x/2) = sinx
4. cos4x + 4cos2x + 3 = 8cos^4(x)
5. 1+cosx+cos2x+cos3x/ 2cos^2(x)+cosx-1 = 2cosx
\(sin^8x-cos^8x-4sin^6x+6sin^4x-4sin^2x\)
\(=sin^8x-\left(1-sin^2x\right)^4-4sin^6x+6sin^4x-4sin^2x\)
\(=sin^8x-\left(1-4sin^2x+6sin^4x-4sin^6x+sin^8x\right)-4sin^6x+6sin^4x-4sin^2x\)\(=-1\) (bạn chép nhầm đề)
b/ \(\frac{sin6x+sin2x+sin4x}{1+cos2x+cos4x}=\frac{2sin4x.cos2x+sin4x}{1+cos2x+2cos^22x-1}=\frac{sin4x\left(2cos2x+1\right)}{cos2x\left(2cos2x+1\right)}=\frac{sin4x}{cos2x}=\frac{2sin2x.cos2x}{cos2x}=2sin2x\)
c/ \(\frac{1+sin2x}{cosx+sinx}-\frac{1-tan^2\frac{x}{2}}{1+tan^2\frac{x}{2}}=\frac{sin^2x+cos^2x+2sinx.cosx}{cosx+sinx}-\left(1-tan^2\frac{x}{2}\right)cos^2\frac{x}{2}\)
\(=\frac{\left(sinx+cosx\right)^2}{sinx+cosx}-\left(cos^2\frac{x}{2}-sin^2\frac{x}{2}\right)=sinx+cosx-cosx=sinx\)
d/ \(cos4x+4cos2x+3=2cos^22x-1+4cos2x+3\)
\(=2\left(cos^22x+2cos2x+1\right)=2\left(cos2x+1\right)^2=2\left(2cos^2x-1+1\right)^2=8cos^4x\)
e/
Đúng 0
Bình luận (1)
Giải các phương trình sau:
1. tan2x+3= (1+√2 sin x)(tan x+ √2 cos x)
2. (1- cos x. cos2x )/ sin2x - 1/ cos x= 4 sin2x - sin x-1
3. sin3x + 2 cos3x+ cos2x - 2sin2x - 2sinx-1=0
1)cos2x+52sqrt{2}left(2-cosxright)sinleft(x-frac{pi}{4}right)
2)
sin^2x-2sinx+2sin^23x
3)
sinx-2sin2x-sin3x2sqrt{2}
4)
left(cos4x-cos2xright)^25+sin3x
5)
sqrt{5+sin^23xsinx+2cosx}
6)
5left(sinx+frac{cos3x+sin3x}{1+2sin2x}right)cos2x+3
7)
frac{sin^42x+cos^42x}{tanleft(frac{pi}{4}-xright)tanleft(frac{pi}{4}+xright)}cos^44x
Đọc tiếp
1)\(cos2x+5=2\sqrt{2}\left(2-cosx\right)sin\left(x-\frac{\pi}{4}\right)\)
2)
\(sin^2x-2sinx+2=sin^23x\)
3)
\(sinx-2sin2x-sin3x=2\sqrt{2}\)
4)
\(\left(cos4x-cos2x\right)^2=5+sin3x\)
5)
\(\sqrt{5+sin^23x=sinx+2cosx}\)
6)
\(5\left(sinx+\frac{cos3x+sin3x}{1+2sin2x}\right)=cos2x+3\)
7)
\(\frac{sin^42x+cos^42x}{tan\left(\frac{\pi}{4}-x\right)tan\left(\frac{\pi}{4}+x\right)}=cos^44x\)
7.
ĐKXĐ: \(\left\{{}\begin{matrix}sin\left(\frac{\pi}{4}-x\right).sin\left(\frac{\pi}{4}+x\right)\ne0\\cos\left(\frac{\pi}{4}-x\right)cos\left(\frac{\pi}{4}+x\right)\ne0\end{matrix}\right.\)
\(\Leftrightarrow cos2x\ne0\)
Phương trình tương đương:
\(\Leftrightarrow\frac{sin^42x+cos^42x}{tan\left(\frac{\pi}{4}-x\right).cot\left(\frac{\pi}{2}-\frac{\pi}{4}-x\right)}=cos^44x\)
\(\Leftrightarrow\frac{sin^42x+cos^42x}{tan\left(\frac{\pi}{4}-x\right).cot\left(\frac{\pi}{4}-x\right)}=cos^24x\)
\(\Leftrightarrow sin^42x+cos^42x=cos^44x\)
\(\Leftrightarrow\left(sin^22x+cos^22x\right)^2-2sin^22x.cos^22x=cos^44x\)
\(\Leftrightarrow1-\frac{1}{2}sin^24x=cos^44x\)
\(\Leftrightarrow2-\left(1-cos^24x\right)=2cos^44x\)
\(\Leftrightarrow2cos^44x-cos^24x-1=0\)
\(\Leftrightarrow\left(cos^24x-1\right)\left(2cos^24x+1\right)=0\)
\(\Leftrightarrow cos^24x-1=0\)
\(\Leftrightarrow sin^24x=0\Leftrightarrow sin4x=0\)
\(\Leftrightarrow2sin2x.cos2x=0\Leftrightarrow sin2x=0\)
\(\Leftrightarrow x=\frac{k\pi}{2}\)
1.
\(cos2x+5=2\left(2-cosx\right)\left(sinx-cosx\right)\)
\(\Leftrightarrow2cos^2x+4=4sinx-4cosx-2sinx.cosx+2cos^2x\)
\(\Leftrightarrow2sinx.cosx-4\left(sinx-cosx\right)+4=0\)
Đặt \(sinx-cosx=t\Rightarrow\left\{{}\begin{matrix}\left|t\right|\le\sqrt{2}\\2sinx.cosx=1-t^2\end{matrix}\right.\)
Pt trở thành:
\(1-t^2-4t+4=0\)
\(\Leftrightarrow t^2+4t-5=0\Leftrightarrow\left[{}\begin{matrix}t=1\\t=-5\left(l\right)\end{matrix}\right.\)
\(\Leftrightarrow\sqrt{2}sin\left(x-\frac{\pi}{4}\right)=1\)
\(\Leftrightarrow\left[{}\begin{matrix}x-\frac{\pi}{4}=\frac{\pi}{4}+k2\pi\\x-\frac{\pi}{4}=\frac{3\pi}{4}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{2}+k2\pi\\x=\pi+k2\pi\end{matrix}\right.\)
2.
\(\Leftrightarrow\left(sinx-1\right)^2+1=sin^23x\)
Ta có \(VT\ge1\) trong khi \(VP\le1\) với mọi x
Đẳng thức xảy ra khi và chỉ khi:
\(\left\{{}\begin{matrix}sinx-1=0\\sin^23x=1\end{matrix}\right.\) \(\Leftrightarrow x=\frac{\pi}{2}+k2\pi\)
3.
\(\Leftrightarrow-2cos2x.sinx-2sin2x=2\sqrt{2}\)
\(\Leftrightarrow cos2x.sinx+sin2x=-\sqrt{2}\)
Ta có:
\(VT^2=\left(cos2x.sinx+sin2x.1\right)^2\le\left(cos^22x+sin^22x\right)\left(sin^2x+1\right)\le1\left(1+1\right)=2\)
\(\Rightarrow VT\ge-\sqrt{2}\)
Dấu "=" xảy ra khi và chỉ khi: \(\left\{{}\begin{matrix}sinx=1\\cos2x=sinx.sin2x\end{matrix}\right.\) (ko tồn tại x thỏa mãn)
Vậy pt vô nghiệm
Xem thêm câu trả lời
Tìm m để các bất phương trình
4
sin
2
x
+
cos
2
x
+
17
3
cos
2
x
+
sin
2
x
+
m
+
1...
Đọc tiếp
Tìm m để các bất phương trình 4 sin 2 x + cos 2 x + 17 3 cos 2 x + sin 2 x + m + 1 ≥ 2 đúng với mọi x ∈ R.
A. 10 - 3 < m ≤ 15 - 29 2
B. 10 - 1 < m ≤ 15 - 29 2
C. 10 - 1 < m ≤ 15 + 29 2
D. 10 - 1 < m < 10 + 1
Tìm giá trị lớn nhất M của hàm số
y
4
sin
2
x
+
2
sin
2
x
+
π
4
.
A.
M
2
.
B.
M
2
−
1....
Đọc tiếp
Tìm giá trị lớn nhất M của hàm số y = 4 sin 2 x + 2 sin 2 x + π 4 .
A. M = 2 .
B. M = 2 − 1.
C. M = 2 + 1.
D. M = 2 + 2.
Ta có y = 4 sin 2 x + 2 sin 2 x + π 4 = 4 1 − cos 2 x 2 + sin 2 x + cos 2 x
= sin 2 x − cos 2 x + 2 = 2 sin 2 x − π 4 + 2.
Mà − 1 ≤ sin 2 x − π 4 ≤ 1 ⇒ − 2 + 2 ≤ 2 sin 2 x − π 4 + 2 ≤ 2 + 2
Vậy giá trị lớn nhất của hàm số là 2 + 2 .
Chọn đáp án D.
Đúng 0
Bình luận (0)
Tìm giá trị lớn nhất M của hàm số
y
4
sin
2
x
+
2
sin
2
x
+
π
4
?
Đọc tiếp
Tìm giá trị lớn nhất M của hàm số y = 4 sin 2 x + 2 sin 2 x + π 4 ?
![]()
![]()
![]()
![]()
Tìm giá trị lớn nhất M của hàm số
y
4
sin
2
x
+
2
sin
2
x
+
π
4
.
A.
M
2
.
B.
M
2
−
1.
C.
M
2...
Đọc tiếp
Tìm giá trị lớn nhất M của hàm số y = 4 sin 2 x + 2 sin 2 x + π 4 .
A. M = 2 .
B. M = 2 − 1.
C. M = 2 + 1.
D. M = 2 + 2.
Ta có y = 4 sin 2 x + 2 sin 2 x + π 4 = 4 1 − cos 2 x 2 + sin 2 x + cos 2 x
= sin 2 x − cos 2 x + 2 = 2 sin 2 x − π 4 + 2.
Mà − 1 ≤ sin 2 x − π 4 ≤ 1 ⇒ − 2 + 2 ≤ 2 sin 2 x − π 4 + 2 ≤ 2 + 2
Vậy giá trị lớn nhất của hàm số là 2 + 2 .
Chọn đáp án D.
Đúng 0
Bình luận (0)