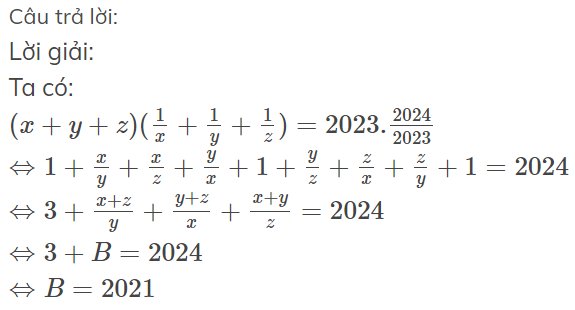1 1/2 x 1 1/3 x 1 1/4 x ....x 1 1/2023 x 1 1/2024

Những câu hỏi liên quan
B=(1+2/1)x(1+2/2)x(1+2/3)x(1+2/4)x....(1+2/2023)x(1+2/2024)
Biết: x + (x - 1) - (x - 2) + (x - 3) - (x - 4) +.....+ (x - 2023) - (x -2024) =0
Vậy x =?
A. 0
B. -1011
C. -1012
D. -2024
\(\dfrac{x-5}{3}=\dfrac{-12}{5-x}\)
\(\dfrac{1}{3}+\dfrac{1}{6}+\dfrac{1}{10}+...+\dfrac{2}{x\left(x+1\right)}=\dfrac{2023}{2024}\)
a
ĐK: \(x\ne5\)
\(\dfrac{x-5}{3}=\dfrac{-12}{5-x}\\ \Leftrightarrow\dfrac{x-5}{3}=\dfrac{12}{x-5}\\ \Leftrightarrow\left(x-5\right)^2=12.3=36\\ \Leftrightarrow\left\{{}\begin{matrix}x-5=6\\x-5=-6\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=11\left(tm\right)\\x=-1\left(tm\right)\end{matrix}\right.\)
b
ĐK: \(x\ne0;x\ne-1\)
\(\dfrac{1}{3}+\dfrac{1}{6}+\dfrac{1}{10}+....+\dfrac{2}{x\left(x+1\right)}=\dfrac{2023}{2024}\)
\(\Leftrightarrow\dfrac{2}{6}+\dfrac{2}{12}+\dfrac{2}{20}+....+\dfrac{2}{x\left(x+1\right)}=\dfrac{2023}{2024}\\ \Leftrightarrow2\left(\dfrac{1}{2.3}+\dfrac{1}{3.4}+\dfrac{1}{4.5}+....+\dfrac{1}{x}.\dfrac{1}{x+1}\right)=\dfrac{2023}{2024}\\ \Leftrightarrow2\left(\dfrac{1}{2}-\dfrac{1}{x+1}\right)=\dfrac{2023}{2024}\\ \Leftrightarrow\dfrac{1}{2}-\dfrac{1}{x+1}=\dfrac{2023}{4048}\\ \Leftrightarrow\dfrac{1}{x+1}=\dfrac{1}{2}-\dfrac{2023}{4048}=\dfrac{1}{4048}\\ \Leftrightarrow4048=x+1\\ \Leftrightarrow x=4047\left(tm\right)\)
Đúng 1
Bình luận (0)
a: =>(x-5)/3=12/(x-5)
=>(x-5)^2=36
=>x-5=6 hoặc x-5=-6
=>x=11 hoặc x=-1
b: =>\(2\left(\dfrac{1}{6}+\dfrac{1}{12}+...+\dfrac{1}{x\left(x+1\right)}\right)=\dfrac{2023}{2024}\)
=>1/2-1/3+1/3-1/4+...+1/x-1/x+1=2023/4048
=>1/2-1/x+1=2023/4048
=>1/(x+1)=1/4048
=>x+1=4048
=>x=4047
Đúng 1
Bình luận (0)
X+1/2020 + X+2/2019=X-1/2023 + X-2/2024
tìm x thuộc z biết x + (x+1) + (x+2) + ... + 2023 + 2024 = 2024
\(x+\left(x+1\right)+\left(x+2\right)+...+2023+2024=2024\)
\(\Rightarrow2023x+4090506=2024-2024-20232023\)
\(\Rightarrow x+4090506=-2023\)
\(\Rightarrow2023x=-2023-4090506\)
\(\Rightarrow2023x=-4092529\)
\(\Rightarrow x=-2023\).
Đúng 2
Bình luận (0)
tìm x:
\(\dfrac{1}{2}+\dfrac{1}{4}+\dfrac{1}{8}+\dfrac{1}{16}+...+\dfrac{1}{x}=\dfrac{2023}{2024}\)
Cho lim x--->-vô cực x.(cănx^2+1)+2023 /(x-2024)=1/2; limx-->+vô cực ((cănx^2+bx+1) -1)=2 tính p=4a+b
Xem chi tiết
Em ghi đề bằng latex đi, thế này ko dịch ra được
Đúng 0
Bình luận (0)
Cho x+y+z=2023 và 1/x + 1/y + 1/z = 2024/2023 Tính giá trị biểu thức B= y+z/x + z+x/y +x+y/z
Lời giải:
Ta có:
$(x+y+z)(\frac{1}{x}+\frac{1}{y}+\frac{1}{z})=2023.\frac{2024}{2023}$
$\Leftrightarrow 1+\frac{x}{y}+\frac{x}{z}+\frac{y}{x}+1+\frac{y}{z}+\frac{z}{x}+\frac{z}{y}+1=2024$
$\Leftrightarrow 3+\frac{x+z}{y}+\frac{y+z}{x}+\frac{x+y}{z}=2024$
$\Leftrightarrow 3+B=2024$
$\Leftrightarrow B=2021$
Đúng 0
Bình luận (0)