ĐK: \(x\ge0\)

Dễ thấy \(1-\sqrt{2\left(x^2-x+1\right)}\le1-\sqrt{2}< 0\)

Khi đó bất phương trình tương đương:

\(x-\sqrt{x}\le1-\sqrt{2\left(x^2-x+1\right)}\)

\(\Leftrightarrow\sqrt{x}-\dfrac{1}{\sqrt{x}}-1+\sqrt{2\left(x+\dfrac{1}{x}-1\right)}\le0\)

\(\Leftrightarrow\sqrt{x}-\dfrac{1}{\sqrt{x}}-1+\sqrt{2\left(\sqrt{x}-\dfrac{1}{\sqrt{x}}\right)^2+2}\le0\)

\(\Leftrightarrow t-1+\sqrt{2t^2+2}\le0\)

\(\Leftrightarrow\dfrac{\left(x-1\right)\left(x-2\right)}{\left(3-x\right)\left(3+x\right)}>0\)

Bảng xét dấu:

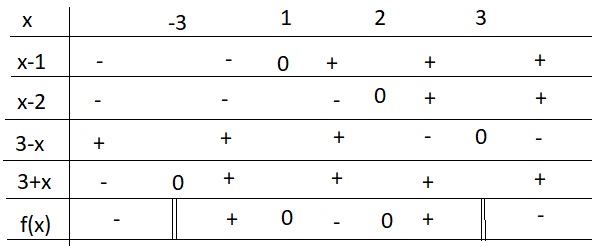
Từ bảng xét dấu ta có nghiệm của BPT là: \(x\in\left(-3;1\right)\cup\left(2;3\right)\)

Do \(x^2+x+1=\left(x+\dfrac{1}{2}\right)^2+\dfrac{3}{4}>0;\forall x\) nên BPT tương đương:

\(-3\left(x^2+x+1\right)\le x^2-3x-1\le3\left(x^2+x+1\right)\)

\(\Leftrightarrow\left[{}\begin{matrix}x^2-3x-1\ge-3x^2-3x-3\\x^2-3x-1\le3x^2+3x+3\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}4x^2\ge-2\left(luôn-đúng\right)\\2x^2+6x+4\ge0\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}x\ge-1\\x\le-2\end{matrix}\right.\)

=>\(\dfrac{3x+5}{3}>\dfrac{-4}{6x+5}\)

=>\(\dfrac{18x^2+15x+30x+25+12}{3\left(6x+5\right)}>0\)

=>\(\dfrac{18x^2+45x+37}{3\left(6x+5\right)}>0\)

=>6x+5>0

=>x>-5/6

\(\left|4x-1\right|=5-x\)

\(\Leftrightarrow\left[{}\begin{matrix}4x-1=5-x\left(x\ge\dfrac{1}{4}\right)\\4x-1=x-5\left(x< \dfrac{1}{4}\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{6}{5}\left(nhận\right)\\x=-\dfrac{4}{3}\left(nhận\right)\end{matrix}\right.\)

\(\left(a-1\right)x+2a+1>0\)

=>\(\left(a-1\right)x>-2a-1\)

=>\(x>\dfrac{-2a-1}{a-1}\)

a: =>3x+3=4x-4

=>-x=-7

hay x=7(nhận)

b: (x-1)(x-3)=0

=>x-1=0 hoặc x-3=0

=>x=1 hoặc x=3

c: 2(x-1)+x=0

=>2x-2+x=0

=>3x-2=0

hay x=2/3

a, ĐKXĐ : x ≠ 1 ; x ≠ -1

\(\Rightarrow3\left(x+1\right)=4\left(x-1\right)\)

\(\Leftrightarrow3x+3=4x-4\)

\(\Leftrightarrow-x=-7\)

\(\Leftrightarrow x=7\left(N\right)\)

b,

\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=3\end{matrix}\right.\)

c,

\(\Leftrightarrow2x-2+x=0\)

\(\Leftrightarrow3x=2\)

\(\Leftrightarrow x=\dfrac{2}{3}\)

\(\sqrt{x+3}-\sqrt{7-x}>\sqrt{2x-8}\)

⇔ \(\sqrt{x+3}>\sqrt{7-x}+\sqrt{2x-8}\)

⇔ \(\left\{{}\begin{matrix}4\le x\le8\\x+3>7-x+2x-8+2\sqrt{\left(7-x\right)\left(2x-8\right)}\end{matrix}\right.\)

⇔ \(\left\{{}\begin{matrix}4\le x\le8\\x+3>x-1+2\sqrt{\left(7-x\right)\left(2x+8\right)}\end{matrix}\right.\)

⇔ \(\left\{{}\begin{matrix}4\le x\le8\\4>2\sqrt{\left(7-x\right)\left(2x+8\right)}\end{matrix}\right.\)

⇔ \(\left\{{}\begin{matrix}4\le x\le8\\\sqrt{\left(7-x\right)\left(2x-8\right)}< 2\end{matrix}\right.\)

⇔ \(\left\{{}\begin{matrix}4\le x\le8\\-2x^2+22x-56< 2\end{matrix}\right.\)

⇔ \(\left\{{}\begin{matrix}4\le x\le8\\\left[{}\begin{matrix}x>\dfrac{11+\sqrt{5}}{2}\\x< \dfrac{11-\sqrt{5}}{2}\end{matrix}\right.\end{matrix}\right.\)

⇔ \(\left[{}\begin{matrix}4\le x< \dfrac{11-\sqrt{5}}{2}\\\dfrac{11+\sqrt{5}}{2}< x\le8\end{matrix}\right.\)

Các giá trị nguyên của x thỏa mãn là S = {4 ; 7 ; 8}

Ấy chết sai điều kiện XĐ rồi, bạn sửa lại điều kiện thôi nhé

e: \(\dfrac{12-3x}{2x+6}-3>0\)

\(\Leftrightarrow\dfrac{12-3x-6x-18}{2x+6}>0\)

\(\Leftrightarrow\dfrac{-9x+6}{2x+6}>0\)

\(\Leftrightarrow\dfrac{3x-2}{x+3}< 0\)

=>-3<x<2/3