(5x-1)(5x+1)+4-25x2+6x

Những câu hỏi liên quan
Tìm x:
1. ( 4x4 + 3x3 ) : ( -x3) + ( 15x2 + 6x ) : 3x = 0
2. ( 25x2 - 10x) : ( -5x) - 3( x-2) = 4
3. ( 42x3 - 12x ) : ( -6x) + 7x ( x+2) = 8
`1)<=> -4x-3 + 5x+ 2 =0`
`<=> 5x-4x = -2+3`
`<=> x =1`
`2)<=> -5x +2-3x+6 =4`
`<=> -5x-3x = 4-6-2`
`<=> -8x=-4`
`<=> x=1/2`
`3) <=> -7x^2 +2 +7x^2 +14x =8`
`<=> 14x +2 =8`
`<=> 14x = 6`
`<=> x=3/7`
Đúng 3
Bình luận (0)
Tìm x:
a) (x+2)(x2-2x+4)=35
b) (25x2+5x+1)(5x-1)=-9
a) Ta có: \(\left(x+2\right)\left(x^2-2x+4\right)=35\)
\(\Leftrightarrow x^3+8=35\)
\(\Leftrightarrow x^3=27\)
hay x=3
b) Ta có: \(\left(25x^2+5x+1\right)\left(5x-1\right)=-9\)
\(\Leftrightarrow125x^3-1=-9\)
\(\Leftrightarrow125x^3=-8\)
\(\Leftrightarrow x=-\dfrac{2}{5}\)
Đúng 2
Bình luận (0)
Tìm x:
1) ( 4x3 + 3x3) : x3+ ( 15x2 + 6x) : ( -3x) = 0
2) ( 25x2 - 10x) : 5x + 3 ( x - 2 ) = 4
3) ( 3x + 1 )2 - ( 2x + 1/2 ) 2 = 00
4) x2 + 8x + 16 = 0
5) 25 - 10x + x2 = 0
`1,(4x^3+3x^3):x^3+(15x^2+6x):(-3x)=0`
`<=> 4 + 3 + (-5x) + (-2)=0`
`<=> -5x+5=0`
`<=>-5x=-5`
`<=>x=1`
`2,(25x^2-10x):5x +3(x-2)=4`
`<=> 5x - 2 + 3x-6=4`
`<=> 8x -8=4`
`<=> 8x=12`
`<=>x=12/8`
`<=>x=3/2`
`3,(3x+1)^2-(2x+1/2)^2=0`
`<=> [(3x+1)-(2x+1/2)][(3x+1)+(2x+1/2)]=0`
`<=>( 3x+1-2x-1/2)(3x+1+2x+1/2)=0`
`<=>( x+1/2) (5x+3/2)=0`
`@ TH1`
`x+1/2=0`
`<=>x=0-1/2`
`<=>x=-1/2`
` @TH2`
`5x+3/2=0`
`<=> 5x=-3/2`
`<=>x=-3/2 : 5`
`<=>x=-15/2`
`4, x^2+8x+16=0`
`<=>(x+4)^2=0`
`<=>x+4=0`
`<=>x=-4`
`5, 25-10x+x^2=0`
`<=> (5-x)^2=0`
`<=>5-x=0`
`<=>x=5`
Đúng 3
Bình luận (1)
rút gọn các biểu thức sau
a) (5x-1)2+(5x+1)2-2(1-25x2)
(5x-1)2+(5x+1)2-2(1-25x2)=25x2-10x+1+25x2+10x+1-2-50x2
= 0
Đúng 0
Bình luận (0)
Lời giải:
$(5x-1)^2+(5x+1)^2-2(1-25x^2)$
$=(5x-1)^2+(5x+1)^2-2(1-5x)(1+5x)$
$=(5x-1)^2+(5x+1)^2+2(5x-1)(5x+1)$
$=(5x-1+5x+1)^2$
$=(10x)^2=100x^2$
Đúng 0
Bình luận (0)
Ta có : \(\left(5x-1\right)^2-2\left(1-25x^2\right)+\left(5x+1\right)^2\)
\(=\left(5x-1\right)^2+2\left(25x^2-1\right)+\left(5x+1\right)^2\)
\(=\left(5x-1\right)^2+2\left(5x-1\right)\left(5x+1\right)+\left(5x+1\right)^2\)
\(=\left(5x-1+5x+1\right)^2=\left(10x\right)^2=100x^2\)
Đúng 0
Bình luận (0)
Phương trình
2
2
x
2
-
5
x
+
2
+
2
3
x
2
-
7
x
+
2
1
+
2...
Đọc tiếp
Phương trình 2 2 x 2 - 5 x + 2 + 2 3 x 2 - 7 x + 2 = 1 + 2 5 x 2 - 12 x + 4 có bao nhiêu nghiệm?
A. 1
B. 2
C. 4
D. 3
Chọn D.
Đặt ![]()
Ta có a + b = 1 + ab ![]()
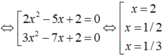
Có 3 nghiệm
Đúng 0
Bình luận (0)
Bài 3: Tìm x
a) (2x+3)2−4x2=10
b) (x+1)2−(2+x)(x−2)=0
c) (5x−1)(1+5x)=25x2−7x+15
d) (4−x)2−16=0
e) 3x2−12x=0
g) x2−8x−3x+24=0
e: \(\Leftrightarrow3x\left(x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=4\end{matrix}\right.\)
Đúng 1
Bình luận (0)
Bài 1: Rút Gọn
a)(x+7)(x-7)-x2
b)(5x-1)(5x+1)-(25x2+1)
c)(4x+1)(4x-1)-(4x-1)2
d)(3x-5)2-(3x+5)(3x-5)
a) \(=x^2-49-x^2\) \(=-49\)
b) \(=25x^2-1-25x^2-1\) \(=-2\)
c) \(=16x^2-1-16x^2+8x-1\) \(=8x-2\)
d) \(=9x^2-30x+25-9x^2+25\) \(=50-30x\)
Đúng 0
Bình luận (0)
Thực hiện phép tính:
1)(x3-8):(x-2)
2)(x3-1):(x2+x+1)
3)(x3+3x2+3x+1):(x2+2x+1)
4)(25x2-4y2):(5x-2y)
1) \(\left(x^3-8\right):\left(x-2\right)=\left[\left(x-2\right)\left(x^2+2x+4\right)\right]:\left(x-2\right)=x^2+2x+4\)
2) \(\left(x^3-1\right):\left(x^2+x+1\right)=\left[\left(x-1\right)\left(x^2+x+1\right)\right]:\left(x^2+x+1\right)=x-1\)
3) \(\left(x^3+3x^2+3x+1\right):\left(x^2+2x+1\right)=\left(x+1\right)^3:\left(x+1\right)^2=x+1\)
4) \(\left(25x^2-4y^2\right):\left(5x-2y\right)=\left[\left(5x-2y\right)\left(5x+2y\right)\right]:\left(5x-2y\right)=5x+2y\)
Đúng 1
Bình luận (0)
a, (4x+1)(x-3)-(x-7)(4x-1)=15
b, (x+1)(x2-x+1)-x(x2-3)=4
c, (x-3)(x2+3x+9)+x(5-x2)=6x
d, (5x-1)(5x+1)=25x2-7x+15
\(a,\left(4x+1\right)\left(x-3\right)-\left(x-7\right)\left(4x-1\right)=15\\ \Leftrightarrow4x^2+x-12x-3-\left(4x^2-28x-x+7\right)-15=0\\ \Leftrightarrow4x^2-11x-3-4x^2+29x-7-15=0\\ \Leftrightarrow18x=25\\ \Leftrightarrow x=\dfrac{25}{18}\)
Vậy \(x=\dfrac{25}{18}\)
\(b,\left(x+1\right)\left(x^2-x+1\right)-x\left(x^2-3\right)=4\\ \Leftrightarrow x^3+1-x^3+3x-4=0\\ \Leftrightarrow3x-3=0\\ \Leftrightarrow x=1\)
Vậy \(x=1\)
\(c,\left(x-3\right)\left(x^2+3x+9\right)+x\left(5-x^2\right)-6x=0\\ \Leftrightarrow x^3-27+5x-x^3-6x=0\\ \Leftrightarrow-x-27=0\\ \Leftrightarrow x=-27\)
Vậy \(x=-27\)
\(d,\left(5x-1\right)\left(5x+1\right)=25x^2-7x+15\\ \Leftrightarrow25x^2-1-25x^2+7x-15=0\\ \Leftrightarrow7x-16=0\\ \Leftrightarrow x=\dfrac{16}{7}\)
Vậy \(x=\dfrac{16}{7}\)
Đúng 4
Bình luận (0)
phân tích đa thức
x^4+6x^3+11x^2+6x+1
x^4+x^3+x^2+x+1
6x^4+5x^3-38x^2+5x+6
x^4+5x^3-12x^2+5x+1
a)\(x^4+6x^3+11x^2+6x+1\)
\(=x^4+9x^2+1+6x^3+6x+2x^2\)
\(=\left(x^2+3x+1\right)^2\)
Đúng 0
Bình luận (0)
\(x^4+5x^3-12x^2+5x+1\)
\(=\left(x^4-2x^3+x^2\right)+\left(7x^3-14x^2+7x\right)+\left(x^2-2x+1\right)\)
\(=x^2\left(x^2-2x+1\right)+7x\left(x^2-2x+1\right)+\left(x^2-2x+1\right)\)
\(=\left(x^2+7x+1\right)\left(x^2-2x+1\right)\)
\(=\left(x^2+7x+1\right)\left(x-1\right)^2\)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời



























