Giúp e với ạ
Hình bên dưới
Giúp em viết một bài cách làm xôi gấc bằng tiếng anh với ạ , cách trình bằng như hình bên dưới . Giúp em với ạ , em đang cần gấp 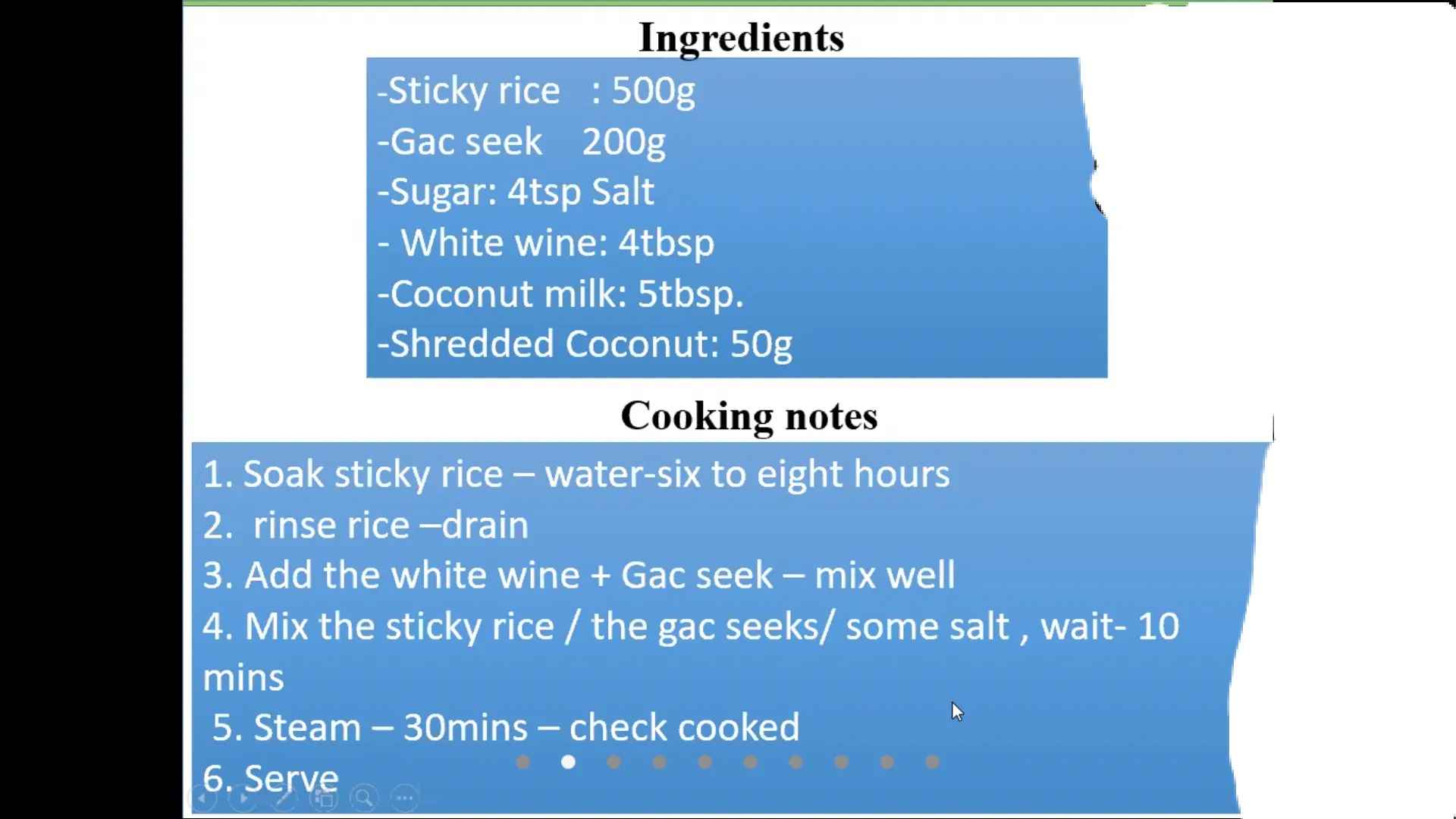

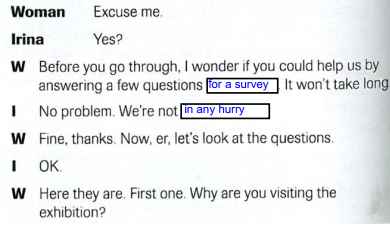
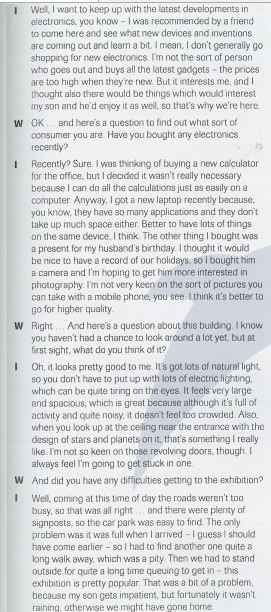
các cao nhân ơi , các cao nhân giúp e với ạ , mọi người chỉ cần làm bài trên ảnh đầu tiên thôi ạ , còn phần bên dưới là script , tại vì mình k có link nghe nên là mk đã cap lại script mọi ng giúp e vs ạ
Chọn 1 trong 4 hình bên dưới rồi vẽ thêm 1 hình chiếu nữa cho đủ ba hình chiếu: đứng, bằng, cạch.
(Giúp e với please)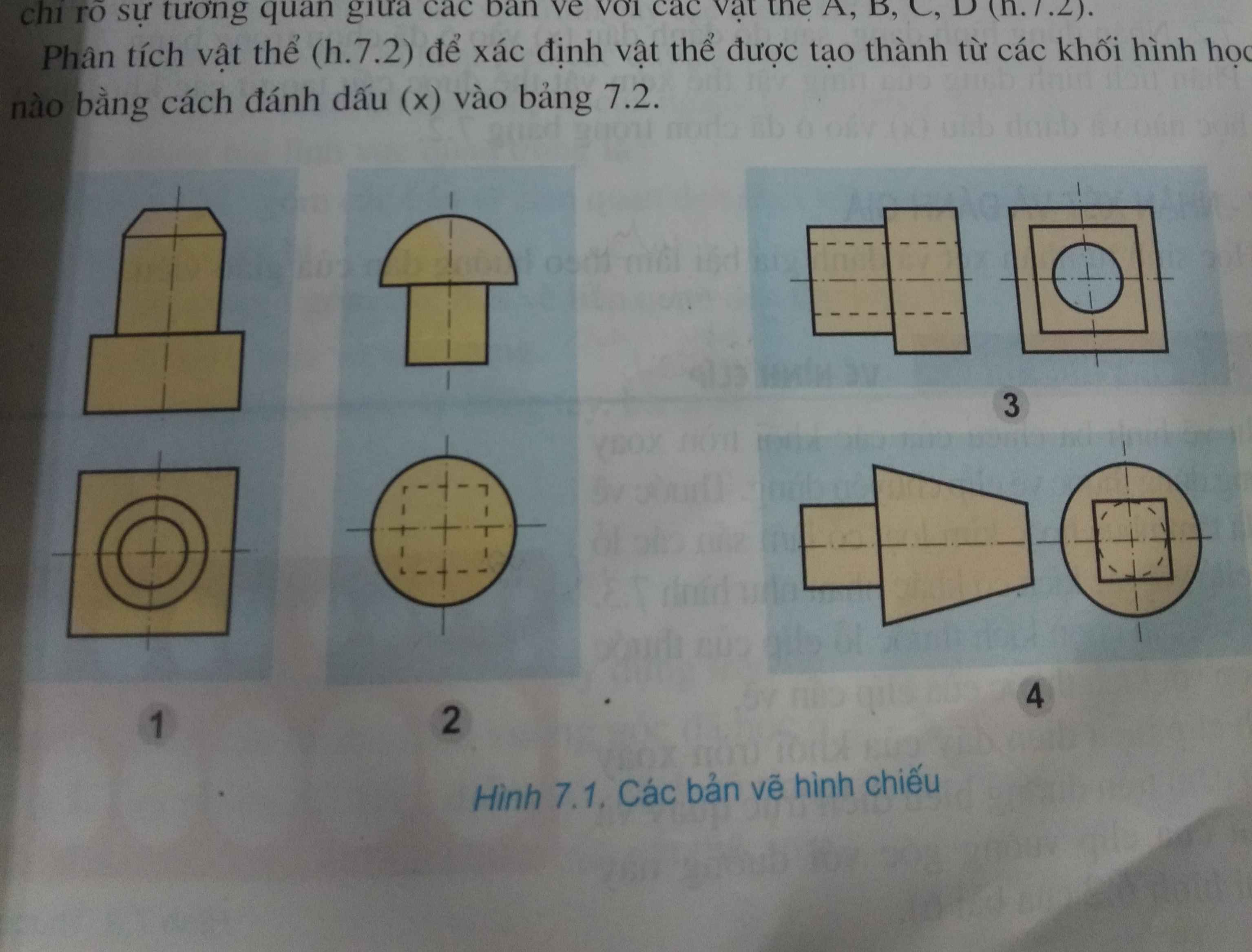
III.
1D 2B 3C 4A 5D 6A 7D 8D
IV.
1, have never met
2, were dancing
3, will die
4, is not going to be built
VI.
1, He studied in Russia.
2, He started his business in the 1990s.
3, He turned some investments into non-profit ventures in healthcare, education and sports.
4, Yes, it is.
VII.
1, Speaking English fluently is very useful.
2, Unless she hurries, she'll miss the last train.
3, A new computer has been bought by my father.
4, Although the weather was bad, they had a picnic.
After a decade of waiting, the Vietnamese football team once again stepped up to take the the AFF Suzuki Cup, as coach Park Hang-seo’s side defeated Malaysian 1-0 in the final second leg at Hanoi’s My Dinh on December 15 evening to win 3-2 on aggregate and be crowned champions in a convincing manner. The impressive journey by the red-shirted men received much praise from the international media.
Right after the game at My Dinh Stadium last night, FOX Sports Asia ran an article pointing out the reasons why the Vietnam team are fully deserving winners of the AFF Cup 2018 title. “The Golden Dragons were excellent throughout their AFF Suzuki Cup campaign, and in the end, lifted the trophy,” it said, adding that the simple reason behind their success was that how to knew play together.
a/ \(\lim\limits_{x\rightarrow3}\dfrac{\sqrt[3]{x^2-1}-2}{x-3}+\lim\limits_{x\rightarrow3}\dfrac{2-\sqrt[4]{1+5x}}{x-3}\)
\(=\lim\limits_{x\rightarrow3}\dfrac{x^2-1-8}{\left(x-3\right)\left(\sqrt[3]{\left(x^2-1\right)^2}+2.\sqrt[3]{x^2-1}+4\right)}+\lim\limits_{x\rightarrow3}\dfrac{16-1-5x}{\left(x-3\right)\left(\sqrt[4]{\left(1+5x\right)^3}+2\sqrt[3]{\left(1+5x\right)^2}+4.\sqrt[3]{1+5x}+8\right)}\)
\(=\lim\limits_{x\rightarrow3}\dfrac{\left(x-3\right)\left(x+3\right)}{\left(x-3\right)\left(\sqrt[3]{\left(x^2-1\right)^2}+2.\sqrt[3]{x^2-1}+4\right)}+\lim\limits_{x\rightarrow3}\dfrac{-5\left(x-3\right)}{\left(x-3\right)\left(\sqrt[4]{\left(1+5x\right)^3}+2\sqrt[3]{\left(1+5x\right)^2}+4\sqrt[3]{1+5x}+8\right)}\)
\(=\dfrac{3+3}{\sqrt[3]{\left(3^2-1\right)^2}+2.\sqrt[3]{3^2-1}+4}-\dfrac{5}{\sqrt[4]{\left(1+5.3\right)^3}+2\sqrt[3]{\left(1+5.3\right)^2}+4.\sqrt[3]{1+5.3}+8}=\dfrac{11}{32}\)
\(\Rightarrow a^2+b^2=1145\)
40/
\(L=\lim\limits_{x\rightarrow0}\dfrac{af\left(x\right)+b^n-b^n}{f\left(x\right)\left[\sqrt[n]{\left(af\left(x\right)+b^n\right)^{n-1}}+b.\sqrt[n]{\left(af\left(x\right)+b^n\right)^{n-2}}+....+b^{n-1}\right]}\)
\(L=\lim\limits_{x\rightarrow0}\dfrac{a}{\sqrt[n]{\left(af\left(x\right)+b^n\right)^{n-1}}+b.\sqrt[n]{\left(af\left(x\right)+b^n\right)^{n-2}}+...+b^{n-1}}\)
\(L=\lim\limits_{x\rightarrow0}\dfrac{a}{b^{n-1}+b^{n-1}++...+b^{n-1}}=\dfrac{a}{nb^{n-1}}\)
40/
\(\sqrt{1+ax}.\sqrt[3]{1+bx}+\sqrt[4]{1+cx}-1=\left(\sqrt{1+ax}-1\right)+\sqrt{1+ax}\left(\sqrt[3]{1+bx}-1\right)+\sqrt{1+ax}.\sqrt[3]{1+bx}.\left(\sqrt[4]{1+cx}-1\right)\)
\(=\lim\limits_{x\rightarrow0}\dfrac{\sqrt{1+ax}-1}{x}+\lim\limits_{x\rightarrow0}\dfrac{\sqrt{1+ax}\left(\sqrt[3]{1+bx}-1\right)}{x}+\lim\limits_{x\rightarrow0}\dfrac{\sqrt{1+ax}.\sqrt[3]{1+bx}\left(\sqrt[4]{1+cx}-1\right)}{x}\)
\(I_1=\lim\limits_{x\rightarrow0}\dfrac{1+ax-1}{x\left(\sqrt{1+ax}+1\right)}=\dfrac{a}{\sqrt{1+ax}+1}=\dfrac{a}{2}\)
\(I_2=\lim\limits_{x\rightarrow0}\dfrac{\sqrt{1+ax}\left(1+bx-1\right)}{x\left(\sqrt[3]{\left(1+bx\right)^2}+\sqrt[3]{1+bx}+1\right)}=\dfrac{b\sqrt{1+ax}}{\sqrt[3]{\left(1+bx\right)^2+\sqrt[3]{1+bx}+1}}=\dfrac{b}{3}\)
\(I_3=\lim\limits_{x\rightarrow0}\dfrac{\sqrt{1+ax}\sqrt[3]{1+bx}\left(1+cx-1\right)}{x\left(\sqrt[4]{\left(1+cx\right)^3}+\sqrt[3]{\left(1+cx\right)^2}+\sqrt[3]{1+cx}+1\right)}=\dfrac{c}{4}\)
\(\Rightarrow L=\dfrac{a}{2}+\dfrac{b}{3}+\dfrac{c}{4}\)
P/s: Thông cảm mình đang đau đầu nên làm hơi lâu :b
Câu 21. Thực hiện thí nghiệm như hình vẽ dưới
Mọi người giúp e giải chi tiết với ạ
xin lỗi vì đã lm phiền mn ạ, giải hộ em với, em lm hết a với b rồi nên a chị nào giúp em câu c và d với ạ, đc sử dụng dữ kiện của câu trên nhé. GT ( đề bài ) ở bên phải hình vẽ ạ. HTC là hình thag cân ạ, còn cái (n) trong GT là cắt >< Mong mn giúp e ạ
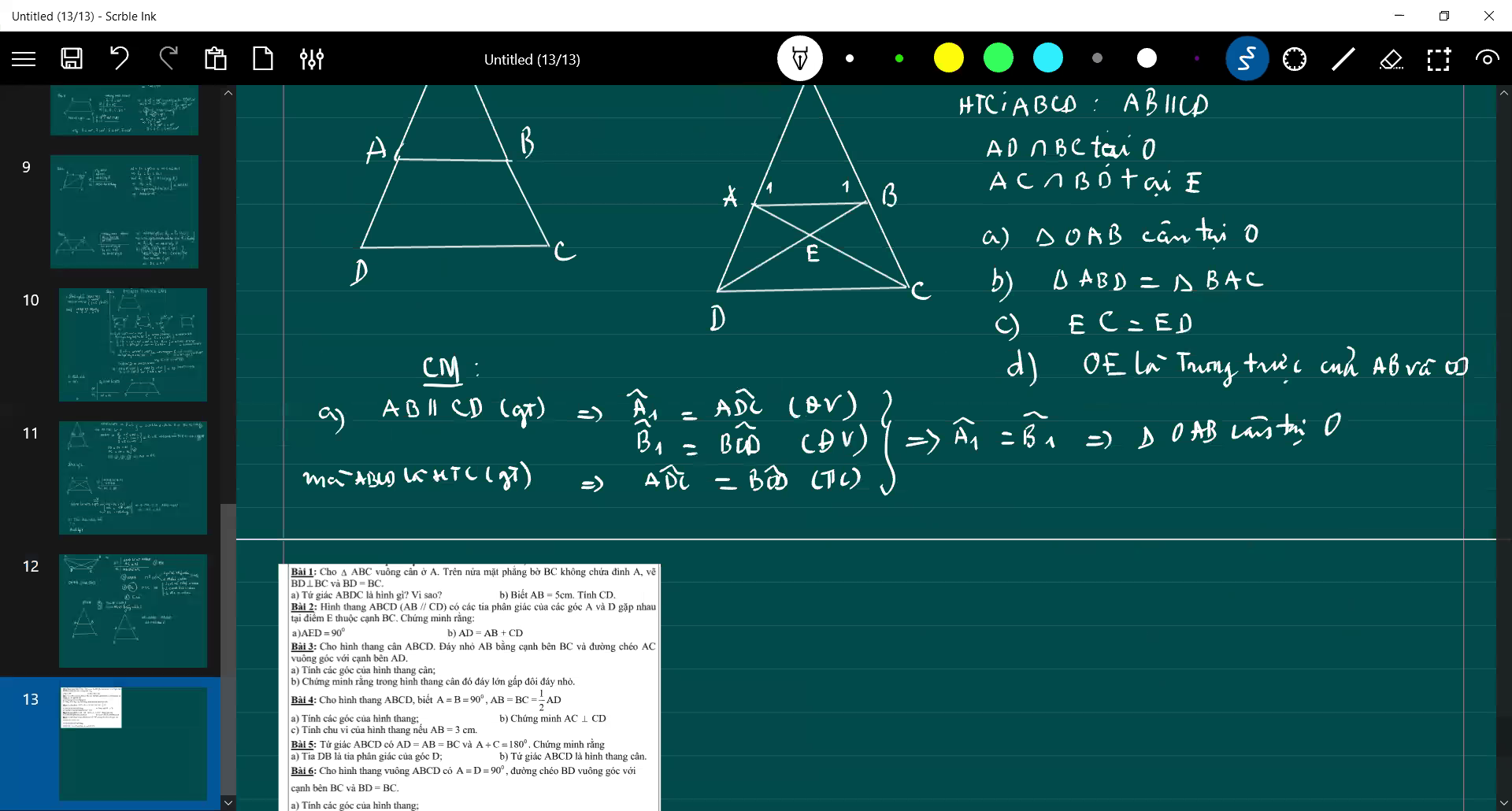
có j thắc mắc thì mn cứ hỏi ạ, em cần trc sáng mai nhé!? ><
b: Xét ΔABD và ΔBAC có
BA chung
BD=AC
AD=BC
Do đó: ΔABD=ΔBAC
c: ta có: EA+EC=AC
EB+ED=BD
mà AC=BD
và EA=EB
nên EC=ED
Giúp e bài này với ạ E đăng ảnh ko được ,nên e để dưới cờ men ạ!e cảm on
"về niêm, hai liên thơ liền nhau được tính theo nguyên tắc: Chứ thứ 2của câu chẵn thuộc liên trên phải cùng thanh với chữ thứ 2 của câu lẻ bên dưới" Mn giải thích cho em câu này với :-(( Mn lấy luôn ví dụ giúp e dễ hiểu ạ
Anh chị ơi giúp em với ạ. Bài tập em sẽ gửi bên dưới mong anh chị giúp em.Nếu đúng em sẽ vote cho anh chị ạ. Em cảm ơn
* Phần bài tập