Giải hệ pt sau
x2 - 2y2 = 2x + y và y2 - 2x2 = 2y + x
1. cho x+y = 1 . tìm GTNN của biểu thức C = x2 + y2
2. cho x + 2y =1 . tìm GTNN của biểu thức P = x2 + 2y2
3. cho x + y =1 . tìm GTNN của biểu thức G = 2x2 + y2
4. cho x + y =1 . tìm GTNN của biểu thức H = x2 + 3y2
5. cho 2x + y =1 . tìm GTNN của biểu thức I = 4x2 + 2y2
6. tìm các số thực thõa mãn Pt :
2x2 + 5y2 + 8x - 10y + 13 = 0
Áp dụng Bunyakovsky, ta có :
\(\left(1+1\right)\left(x^2+y^2\right)\ge\left(x.1+y.1\right)^2=1\)
=> \(\left(x^2+y^2\right)\ge\frac{1}{2}\)
=> \(Min_C=\frac{1}{2}\Leftrightarrow x=y=\frac{1}{2}\)
Mấy cái kia tương tự
Giải hệ pt:hệ bậc 2-2 ẩn
x2+2x-2y2=0
y2+2y-2x2=0
\(\left\{{}\begin{matrix}x^2+2x-2y^2=0\\y^2+2y-2x^2=0\end{matrix}\right.\)\(\left(1\right)-\left(2\right)\Rightarrow x^2+2x-2y^2-y^2-2y+2x^2=0\)
\(\Leftrightarrow\left(x-y\right)\left(3x+3y+2\right)=0\Leftrightarrow\left(x-y\right)3\left(x+y+\dfrac{2}{3}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-y=0\\x+y+\dfrac{2}{3}=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=y\left(2\right)\\x=-\dfrac{2}{3}-y\left(3\right)\end{matrix}\right.\)
\(thế\left(2\right)và\left(3\right)lên-hệ-pt-rồi-giải\)
Giải hệ phương trình 2 x 2 − y 2 + x y − 5 x + y + 2 = y − 2 x + 1 − 3 − 3 x x 2 − y − 1 = 4 x + y + 5 − x + 2 y − 2
ĐK: y − 2 x + 1 ≥ 0 , 4 x + y + 5 ≥ 0 , x + 2 y − 2 ≥ 0 , x ≤ 1
T H 1 : y − 2 x + 1 = 0 3 − 3 x = 0 ⇔ x = 1 y = 1 ⇒ 0 = 0 − 1 = 10 − 1 ( k o t / m ) T H 2 : x ≠ 1 , y ≠ 1
Đưa pt thứ nhất về dạng tích ta được
( x + y − 2 ) ( 2 x − y − 1 ) = x + y − 2 y − 2 x + 1 + 3 − 3 x ( x + y − 2 ) 1 y − 2 x + 1 + 3 − 3 x + y − 2 x + 1 = 0 ⇒ 1 y − 2 x + 1 + 3 − 3 x + y − 2 x + 1 > 0 ⇒ x + y − 2 = 0
Thay y= 2-x vào pt thứ 2 ta được x 2 + x − 3 = 3 x + 7 − 2 − x
⇔ x 2 + x − 2 = 3 x + 7 − 1 + 2 − 2 − x ⇔ ( x + 2 ) ( x − 1 ) = 3 x + 6 3 x + 7 + 1 + 2 + x 2 + 2 − x ⇔ ( x + 2 ) 3 3 x + 7 + 1 + 1 2 + 2 − x + 1 − x = 0
Do x ≤ 1 ⇒ 3 3 x + 7 + 1 + 1 2 + 2 − x + 1 − x > 0
Vậy x + 2 = 0 ⇔ x = − 2 ⇒ y = 4 (t/m)
phân tích đa thức thành nhân tử 2 ẩn :
a) 2x2+xy-y2-x+2y-1
b) 3x2-2xy-y2-10x-2y+3
c) 3x2y-xy2+xy-2y2-3x-9y+5
d) 2x2y2-3xy-2y2+y+1
e) 3x3-12xy2-5x2-4y2+x+1
a)2x^2+xy-y^2-x+2y-1
=2x^2+xy-x-(y-1)^2
=2x^2+x(y-1)-(y-1)^2
=2a^2+ab-b^2 với a=x,b=y-1
=2a^2+2ab-ab-b^2
=(2a-b)(a+b)
=(2x-y+1)(x+y-1)
cho x+y=5
P=3x2-2x+3y2-2y+6xy-100
Q=x3+y3-2x2-2y2+3xy(x+y)-4xy+3(x+y)+10
a) \(P=3\left(x^2+2xy+y^2\right)-2\left(x+y\right)-100\)
\(P=3\left(x+y\right)^2-2.5-100\)
\(P=3.5^2-110\)
\(P=-35\)
b) \(Q=\left[x^3+y^3+3xy\left(x+y\right)\right]-2\left(x^2+2xy+y^2\right)+3.5+10\)
\(Q=\left(x+y\right)^3-2\left(x+y\right)^2+25\)
\(Q=5^3-2.5^2+25\)
\(Q=100\)
tìm GTNN của các bt
a, A=2x2+y2-2xy-2x+3
b,B=x2-2xy+2y2+2x-10y+17
c,C=x2-xy+y2-2y-2x
d,D=x2+xy+y2-3y-3x
e,E=2x2+2xy +5y2-8x-22y
A= 2x^2 + y^2 - 2xy -2x+3
A= x^2-2xy + y^2 + x^2 - 2x+ 1 +2
A= (x-y)^2 + (x-1)^2 + 2
(x-y)^2> hoặc = 0 với mọi giá trị của x
(x-1)^2 > hoặc =0 với mọi giá trị của x
=> (x-y)^2 + (x-1)^2 > hoặc =0 với mọi giá trị của x
=> (x-y)^2 + (x-1)^2 + 2 > hoặc =2
=> A lớn hơn hoặc bằng 2
=> GTNN của A=2 tại x=y=1
Rút gọn biểu thức
a. 2x+2y/a2+2ab+b2 . ax-ay+bx-by/2x2-2y2
b. a+b-c/a2+2ab+b2-c2 . a2+2ab+b2+ac+bc/a2-b2
c.x3+1/x2+2x+1 . x2-1/2x2-2x+2
d. x8-1/x+1 . 1/ (x2+1) (x4+1)
e. x-y/xy+y2 - 3x+y/x2-xy . y-x/x+y
a2 c2... là em viết số mũ đó ạ. anh chị giúp em giải mấy bài này nha
\(=\dfrac{2\left(x+y\right)}{\left(a+b\right)^2}.\dfrac{a\left(x-y\right)+b\left(x-y\right)}{2\left(x^2-y^2\right)}\)
\(=\dfrac{2\left(x+y\right)}{\left(a+b\right)^2}.\dfrac{\left(x-y\right)\left(a+b\right)}{2\left(x-y\right)\left(x+y\right)}\)
\(=\dfrac{1}{a+b}\)
\(=\dfrac{a+b-c}{\left(a+b\right)^2-c^2}.\dfrac{\left(a+b\right)^2+c\left(a+b\right)}{\left(a-b\right)\left(a+b\right)}\)
\(=\dfrac{a+b-c}{\left(a+b-c\right)\left(a+b+c\right)}.\dfrac{\left(a+b\right)\left(a+b+c\right)}{\left(a-b\right)\left(a+b\right)}\)
\(=\dfrac{1}{a-b}\)
\(c,\dfrac{x^3+1}{x^2+2x+1}.\dfrac{x^2-1}{2x^2-2x+2}\)
\(=\dfrac{\left(x+1\right)\left(x^2-x+1\right)}{\left(x+1\right)^2}.\dfrac{\left(x-1\right)\left(x+1\right)}{2\left(x^2-x+1\right)}\) \(=\dfrac{x-1}{2}\) \(d,\dfrac{x^8-1}{x+1}.\dfrac{1}{\left(x^2+1\right)\left(x^4+1\right)}\) \(=\dfrac{\left(x^4\right)^2-1}{x+1}.\dfrac{1}{\left(x^2+1\right)\left(x^4+1\right)}\) \(=\dfrac{\left(x^4-1\right)\left(x^4+1\right)}{x+1}.\dfrac{1}{\left(x^2+1\right)\left(x^4+1\right)}\) \(=\dfrac{\left(x^2+1\right)\left(x^2-1\right)}{x+1}.\dfrac{1}{x^2+1}\) \(=\dfrac{\left(x-1\right)\left(x+1\right)}{x+1}\) \(=x-1\) \(e,\dfrac{x-y}{xy+y^2}-\dfrac{3x+y}{x^2-xy}.\dfrac{y-x}{x+y}\) \(=\dfrac{x-y}{y\left(x+y\right)}-\dfrac{3x+y}{x\left(x-y\right)}.\dfrac{-\left(x-y\right)}{x+y}\) \(=\dfrac{x-y}{y\left(x+y\right)}-\dfrac{3x+y}{x}.\dfrac{-1}{x+y}\) \(=\dfrac{x-y}{y\left(x+y\right)}-\dfrac{-3x-y}{x\left(x+y\right)}\) \(=\dfrac{x\left(x-y\right)+y\left(3x+y\right)}{xy\left(x+y\right)}\) \(=\dfrac{x^2-xy+3xy+y^2}{xy\left(x+y\right)}\) \(=\dfrac{x^2+2xy+y^2}{xy\left(x+y\right)}\) \(=\dfrac{\left(x+y\right)^2}{xy\left(x+y\right)}=\dfrac{x+y}{xy}\)Cho hai số thực dương x,y thỏa mãn 2x + 2y = 4. Tìm giá trị lớn nhất Pmax của biểu thức
P = (2x2 + y)(2y2 + x) + 9xy.
A. Pmax = 27 2
B. Pmax = 18
C. Pmax = 27
D. Pmax = 12
Đáp án B.
Ta có 4 = 2 x + 2 y ≥ 2 2 x . 2 y = 2 2 x + y
⇔ 4 ≥ 2 x + y ⇔ x + y ≤ 2 .
Suy ra x y ≤ x + y 2 2 = 1
Khi đó
P = 2 x 3 + y 3 + 4 x 2 y 2 + 10 x y 2 x + y x + y 2 - 3 x y + 2 x y 2 + 10 x y
≤ 4 4 - 3 x y + 4 x 2 y 2 + 10 x y
= 16 + 2 x 2 y 2 + 2 x y x y - 1 ≤ 18
Vậy Pmax = 18 khi x = y = 1.
Giải bất đẳng thức sau
x2+y2 ≥ \(\dfrac{\left(x+y\right)^2}{2}\)≥2xy
Sửa đề:" CM BĐT
x^2+y^2>=(x+y)^2/2
=>2x^2+2y^2-x^2-2xy-y^2>=0
=>(x-y)^2>=0(luôn đúng)(1)
(x+y)^2/2>=2xy
=>(x+y)^2>=4xy
=>(x-y)^2>=0(luôn đúng)(2)
Từ (1), (2) suy ra ĐPCM
Cho hệ phương trình x + y = m - 2 x 2 + y 2 + 2 x + 2 y = - m 2 + 4 (trong đó m là tham số). Tìm tất cả các giá trị của m để hệ có nghiệm
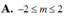
![]()
![]()
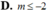
![]()