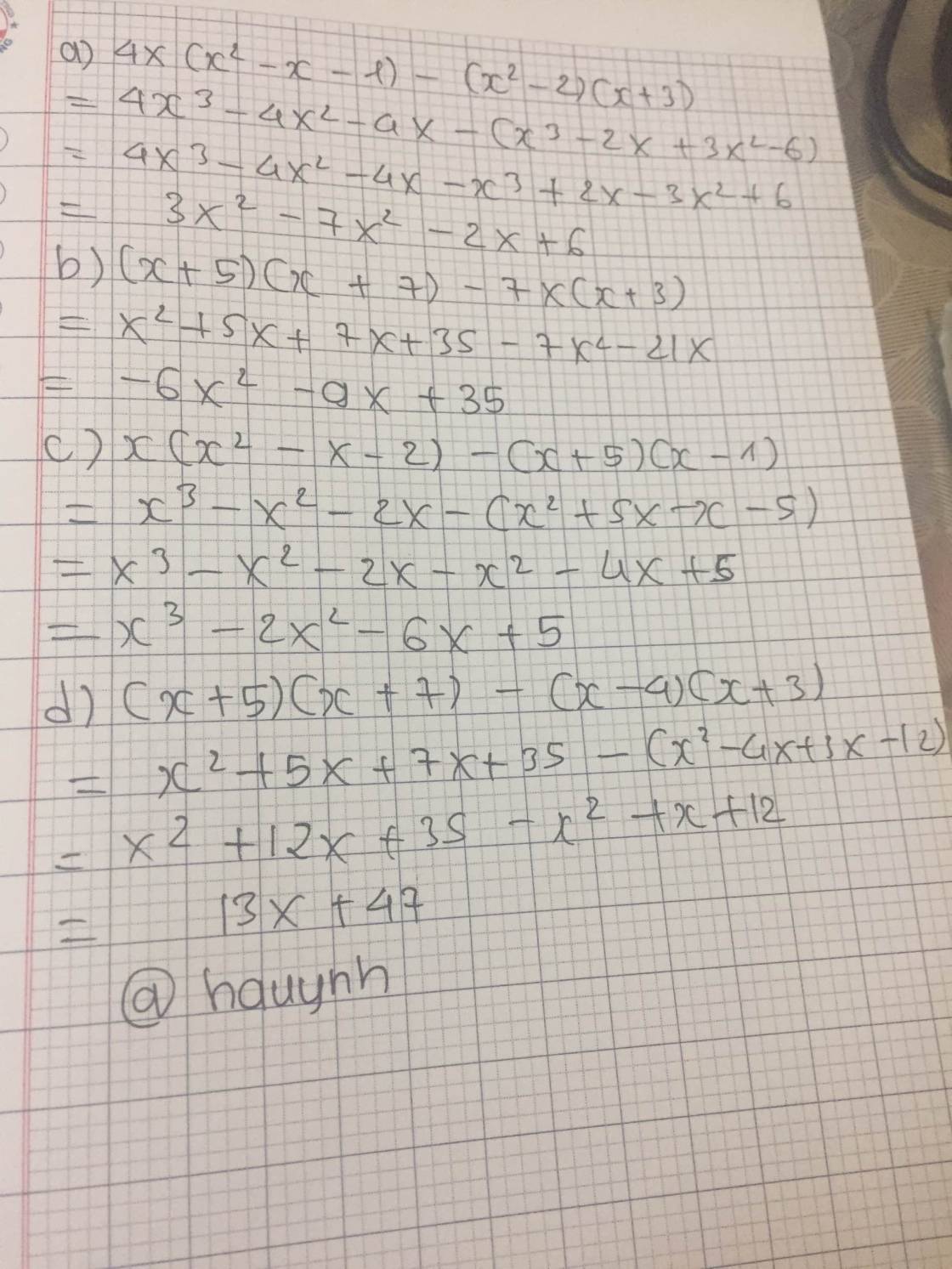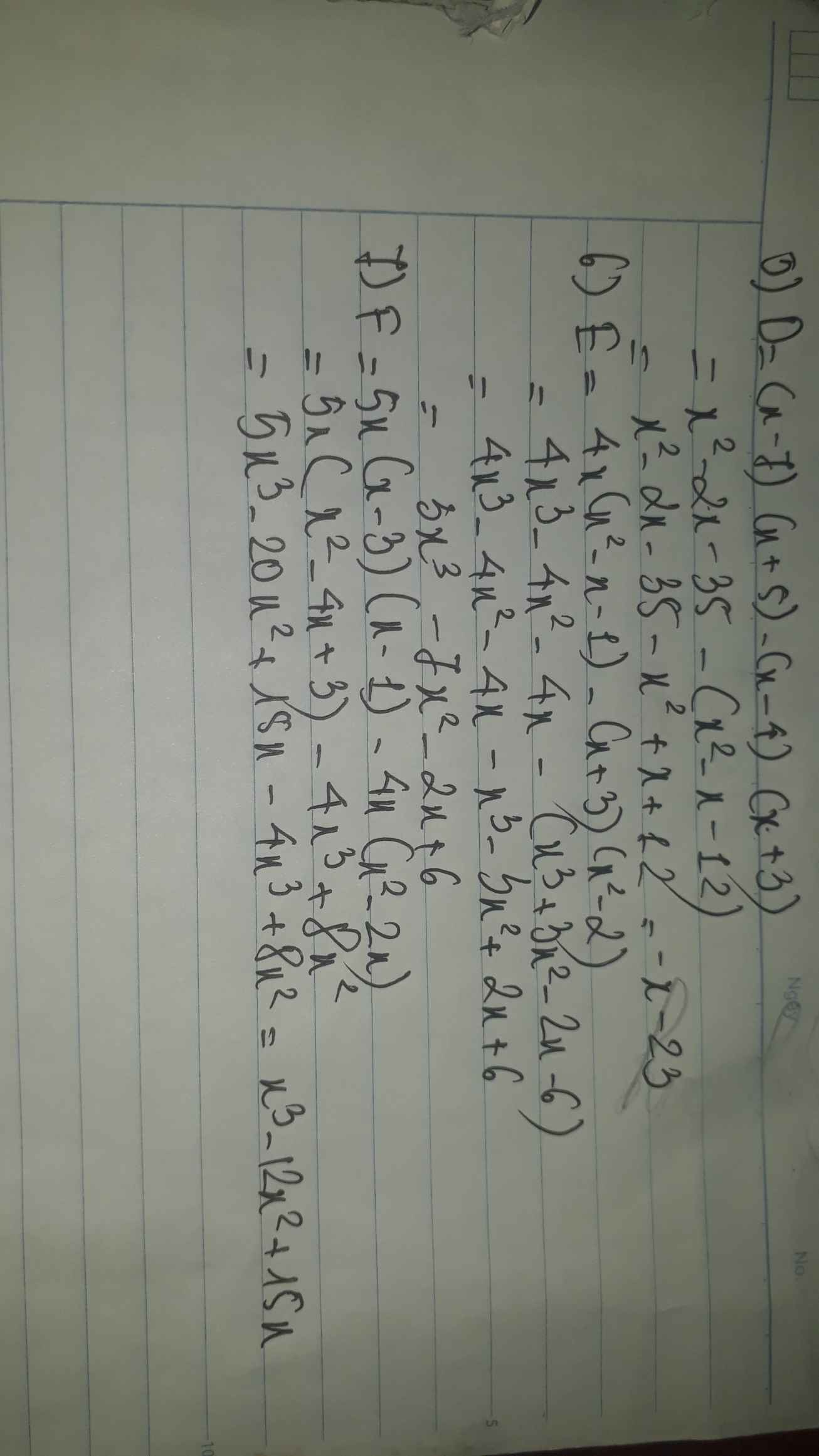2(x+1)√(x+3/x)=x2+7

Những câu hỏi liên quan
1) (\(\dfrac{1}{2}\)x + 3)*(x2- 4x- 6)
2) (6x2 -9x +15)*(\(\dfrac{2}{3}\)x+1)
3) (3x2 -x+5)*(x3+5x-1)
4) (x-1)*(x+1)*(x-2)
5) D=(x-7)*(x+5)-(x-4)*(x+3)
6) E= 4x*(x2-x-1)-(x+3)*(x2-2)
7) F= 5x*(x-3)*(x-1)-4x*(x2-2x)
1) \(\left(\dfrac{1}{2}x+3\right)\left(x^2-4x-6\right)\)
\(=\dfrac{1}{2}x^3-2x^2-3x+3x^2-12x-18\)
\(=\dfrac{1}{2}x^3+x^2-15x-18\)
2) \(\left(6x^2-9x+15\right)\left(\dfrac{2}{3}x+1\right)\)
\(=4x^3+6x^2-6x^2-9x+10x+15\)
\(=4x^3+x+15\)
3) Ta có: \(\left(3x^2-x+5\right)\left(x^3+5x-1\right)\)
\(=3x^5+15x^2-3x^2-x^4-5x^2+x+5x^3+25x-5\)
\(=3x^5-x^4+5x^3+10x^2+26x-5\)
4) Ta có: \(\left(x-1\right)\left(x+1\right)\left(x-2\right)\)
\(=\left(x^2-1\right)\left(x-2\right)\)
\(=x^3-2x^2-x+2\)
Đúng 2
Bình luận (0)
a, 4x(x2-x-1)-(x2-2)(x+3)
b, (x+5)(x+7)-7x(x+3)
c, x(x2-x-2)-(x+5)(x-1)
d, (x+5)(x+7)-(x-4)(x+3)
`@` `\text {dnammv}`
`a,`
`4x(x^2-x-1)-(x^2-2)(x+3)`
`= 4x^3-4x^2-4x- [x^2(x+3)-2(x+3)]`
`= 4x^3-4x^2-4x- (x^3+3x^2-2x-6)`
`= 4x^3-4x^2-4x-x^3-3x^2+2x+6`
`= 3x^3 - 7x^2-2x+6`
`b,`
`(x+5)(x+7)-7x(x+3)`
`= x(x+7)+5(x+7)-7x^2-21x`
`= x^2+7+5x+35-7x^2-21x`
`= -6x^2-16x+35`
`c,`
`x(x^2-x-2)-(x+5)(x-1)`
`= x^3-x^2-2x- [x(x-1)+5(x-1)]`
`= x^3-x^2-2x- (x^2-x+5x-5)`
`= x^3-x^2-2x - x^2 + x -5x+5`
`= x^3-2x^2- 4x+5`
`d,`
`(x+5)(x+7)-(x-4)(x+3)`
`= x(x+7)+5(x+7)- [x(x+3)-4(x+3)]`
`= x^2+7x+5x+35 - (x^2+3x-4x-12)`
`= x^2+12x+35 - x^2+x+12`
`= 13x+47`
Đúng 2
Bình luận (3)
Thực hiện phép tính:
a,4.(x+3)/3x2-x : x2+3x/1-3x
b, x+1/x2-2x-8 . 4-x/x2+x
c, 9x+5/2(x-1)(x+3)2- 5x-7/2(x-1)(x+3)2
d, 18/(x-3)(x2-9)-3/x^2-6x+9-x/x^2-9
e, 1/x2-x+1+1/1-x2+2/x3+1
1) (1-x)(5x+3)=(3x-7)(x-1)
2) (x-2)(x+1)=x2-4
3) 2x3+3x2-32x=48
4) x2+2x-15=0
5) 2x(2x-3)=(3-2x)(2-5x)
6) x3-5x2+6x=0
7) (x2-5)(x+3)=0
8) (x+7)(3x-1)=49-x2
\(\left(1-x\right)\left(5x+3\right)=\left(3x-7\right)\left(x-1\right)\)
\(< =>\left(1-x\right)\left(5x+3+3x-7\right)=0\)
\(< =>\left(1-x\right)\left(8x-4\right)=0\)
\(< =>\orbr{\begin{cases}1-x=0\\8x-4=0\end{cases}< =>\orbr{\begin{cases}x=1\\x=\frac{1}{2}\end{cases}}}\)
\(\left(x-2\right)\left(x+1\right)=x^2-4\)
\(< =>\left(x-2\right)\left(x+1\right)=\left(x-2\right)\left(x+2\right)\)
\(< =>\left(x-2\right)\left(x+1-x-2\right)=0\)
\(< =>-1\left(x-2\right)=0\)
\(< =>2-x=0< =>x=2\)
\(2x^3+3x^2-32x=48\)
\(< =>x^2\left(2x+3\right)-16\left(2x+3\right)=0\)
\(< =>\left(x^2-16\right)\left(2x+3\right)=0\)
\(< =>\left(x-4\right)\left(x+4\right)\left(2x+3\right)=0\)
\(< =>\hept{\begin{cases}x=4\\x=-4\\x=-\frac{3}{2}\end{cases}}\)
Xem thêm câu trả lời
5) D=(x-7)*(x+5)-(x-4)*(x+3)
6) E= 4x*(x2-x-1)-(x+3)*(x2-2)
7) F= 5x*(x-3)*(x-1)-4x*(x2-2x)
Tìm x:
a) x(x+1)(x+2)(x+3) = (x2+3x+1)2+x
b) (x+1)(x+3)(x+5)(x+7) = (x2+8x+11)2+2x
c) (x2-x+1)(x2+x+1)(x-1)(x+1)=63
a) \(x\left(x+1\right)\left(x+2\right)\left(x+3\right)=\left(x^2+3x+1\right)^2+x\)
\(\Leftrightarrow\left(x^2+3x\right)\left(x^2+3x+2\right)=\left(x^2+3x+1\right)^2+x\)
\(\Leftrightarrow\left(t-1\right)\left(t+1\right)=t^2+x\) (với \(t=x^2+3x+1\))
\(\Leftrightarrow t^2-1=t^2+x\)
\(\Leftrightarrow x=-1\).
b) \(\left(x+1\right)\left(x+3\right)\left(x+5\right)\left(x+7\right)=\left(x^2+8x+11\right)^2+2x\)
\(\Leftrightarrow\left(x^2+8x+7\right)\left(x^2+8x+15\right)=\left(x^2+8x+11\right)^2+2x\)
\(\Leftrightarrow\left(t-4\right)\left(t+4\right)=t^2+2x\) (với \(t=x^2+8x+11\))
\(\Leftrightarrow t^2-16=t^2+2x\)
\(\Leftrightarrow x=-8\)
c) \(\left(x^2-x+1\right)\left(x^2+x+1\right)\left(x-1\right)\left(x+1\right)=63\)
\(\Leftrightarrow\left(x^3-1\right)\left(x^3+1\right)=63\)
\(\Leftrightarrow x^6-1=63\)
\(\Leftrightarrow x^6=64\)
\(\Leftrightarrow x=\pm2\)
Đúng 4
Bình luận (0)
https://onlinemath.vn/cau-hoi/viet-1-doan-van-tong-phan-hop-khoang-12-cau-phan-tich-kho-tho-thu-2-bai-que-huong-trong-do-su-dung-1-cau-cam-than-vs-cau-ghep-chi-ro.8109170456376 help
Đúng 0
Bình luận (0)
Bài 2: Rút gọn các biểu thức sau:
a.(x-3)(x + 7) – (x +5)(x -1) b. (x + 8)2 – 2(x +8)(x -2) + (x -2)2
c. x2(x – 4)(x + 4) – (x2 + 1)(x2- 1) d. (x+1)(x2 – x + 1) – (x – 1)(x2 +x +1)
\(a,x^2+4x-21-x^2-4x+5=-16\\ b,=\left(x+8-x+2\right)^2=10^2=100\\ c,=x^2\left(x^2-16\right)-\left(x^4-1\right)\\ =x^4-16x^2-x^4+1=1-16x^2\\ d,=x^3+1-x^3+1=2\)
Đúng 2
Bình luận (0)
Bài 2: Rút gọn các biểu thức sau:
a.(x-3)(x + 7) – (x +5)(x -1) b. (x + 8)2 – 2(x +8)(x -2) + (x -2)2
c. x2(x – 4)(x + 4) – (x2 + 1)(x2- 1) d. (x+1)(x2 – x + 1) – (x – 1)(x2 +x +1)
\(a,=x^2+4x-21-x^2-4x+5=-16\\ b,=\left(x+8-x+2\right)^2=10^2=100\\ c,=x^2\left(x^2-16\right)-\left(x^4-1\right)\\ =x^4-16x^2-x^4+1=1-16x^2\\ d,=x^3+1-x^3+1=2\)
Đúng 0
Bình luận (0)
· Câu 7:Phân tích x3(x2 – 1) - (x2 – 1) thành nhân tử ta được:o A. (x + 1)3(x + 1)o B. (x – 1)(x + 1)(x2 + x + 1)o C. (x – 1)2(x + 1)(x2 – x + 1)o D. (x – 1)2(x + 1)(x2 + x + 1)· Câu 8:(x + 3)2 – 25 được phân tích thành nhân tử là:o A. (x – 8)(x – 2)o B. (x – 8)(x + 2)o C. (x + 8)(x + 2)o D. (x + 8)(x – 2)· Câu 9:Giá trị của biểu thức A x2 – y2 + 2y – 1 với x 75; y 26 là:o A. – 5000o B. 5000o C. 6500o D. – 6500· C...
Đọc tiếp
· Câu 7:Phân tích x3(x2 – 1) - (x2 – 1) thành nhân tử ta được:
o A. (x + 1)3(x + 1)
o B. (x – 1)(x + 1)(x2 + x + 1)
o C. (x – 1)2(x + 1)(x2 – x + 1)
o D. (x – 1)2(x + 1)(x2 + x + 1)
· Câu 8:(x + 3)2 – 25 được phân tích thành nhân tử là:
o A. (x – 8)(x – 2)
o B. (x – 8)(x + 2)
o C. (x + 8)(x + 2)
o D. (x + 8)(x – 2)
· Câu 9:
Giá trị của biểu thức A = x2 – y2 + 2y – 1 với x = 75; y = 26 là:
o A. – 5000
o B. 5000
o C. 6500
o D. – 6500
· Câu 10:
Tìm x biết 2x2 – x – 1 = 0 ta được:
o A. x = - 1 hoặc x = -1/2
o B. x = 1 hoặc x = -1/2
o C. x = - 1 hoặc x = 1/2
· Câu 11:
Giá trị của biểu thức 4(x + y)2 – 9(x – y)2 với x = 2; y = 4 là:
o A. 118
o B. 108
o C. 78
o D. 98
· Câu 12:
Đa thức 49(y – 4)2– 9(y + 2)2 được phân tích thành nhân tử là:
o A. 2(5y + 11)(4y – 24)
o B. 2(5y – 11)(4y + 24)
o C. 2(5y – 11)(4y – 34)
o D. 2(5y + 11)(4y + 34)
· Câu 13:
Đa thức 9x6 + 24x3y2 + 16y2 được phân tích thành nhân tử là:
o A. (3x3 – 4y2)2
o B. (3x3 + 4y2)2
o C. (3y3 – 2x2)2
o D. - (3x3 + 4y2)2
· Câu 14:
Đa thức 36 – 12x + x2 được phân tích thành nhân tử là:
o A. (6 – x)2
o B. (6 + x)2
o C. (6 + x)3
o D. (6 – x)3
\(7,D\\ 8,D\\ 9,B\\ 10,B\\ 11,B\\ 12,C\\ 13,B\\ 14,A\)
Đúng 0
Bình luận (0)
h*) (x + 3)(1 – x) > 0
i*) (x2 – 1)(x2 – 4) < 0
k*) (x2 – 20)(x2 – 30) < 0
Bài 4: Tìm các số nguyên x sao cho
a) –3 ⋮ (x – 2)
b) (3x + 7) ⋮ (x – 2)
c*) (x2 + 7x + 2) ⋮ (x + 7)
a, \(\Rightarrow x-2\inƯ\left(-3\right)=\left\{\pm1;\pm3\right\}\)
| x-2 | 1 | -1 | 3 | -3 |
| x | 3 | 1 | 5 | -1 |
b, \(3\left(x-2\right)+13⋮x-2\Rightarrow x-2\inƯ\left(13\right)=\left\{\pm1;\pm13\right\}\)
| x-2 | 1 | -1 | 13 | -13 |
| x | 3 | 1 | 15 | -11 |
c, \(x\left(x+7\right)+2⋮x+7\Rightarrow x+7\inƯ\left(2\right)=\left\{\pm1;\pm2\right\}\)
| x+7 | 1 | -1 | 2 | -2 |
| x | -6 | -8 | -5 | -9 |
Đúng 0
Bình luận (0)