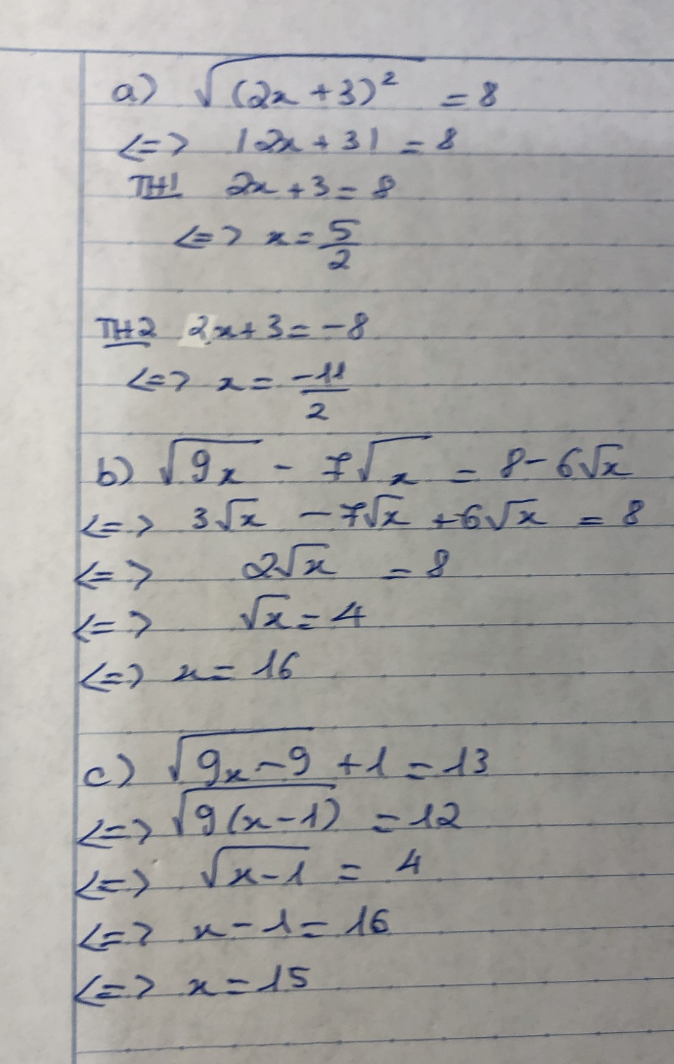Mn giúp e với ak

a) \(\sqrt{x^2-6x+9}\)

\(=\sqrt{\left(x^2-2.x.3+3^2\right)}\)

\(=\sqrt{\left(x-3\right)^2}\) ≥0,∀x

⇒x∈\(R\)

b) \(\sqrt{x^2-2x+1}\)

\(=\sqrt{\left(x^2-2.x.1+1^2\right)}\)

\(=\sqrt{\left(x-1\right)^2}\) ≥0,∀x

⇒x∈\(R\)

Giúp mình với 

Nếu chưa quen giải toán căn thức, em tìm ĐKXĐ cho x, rồi đặt \(\sqrt{x}=t\ge0\Rightarrow x=t^2\) rồi thế vào giải là nó ra 1 pt bình thường theo biến t thôi

a) Ta có: \(\sqrt{9x}+\sqrt{x}=12\)

\(\Leftrightarrow4\sqrt{x}=12\)

\(\Leftrightarrow\sqrt{x}=3\)

hay x=9

b) Ta có: \(\dfrac{\sqrt{x}+3}{4}=\dfrac{\sqrt{x}}{3}\)

\(\Leftrightarrow4\sqrt{x}=3\sqrt{x}+9\)

\(\Leftrightarrow\sqrt{x}=9\)

hay x=81

c) Ta có: \(\dfrac{5\sqrt{x}-x}{\sqrt{x}}=2\)

\(\Leftrightarrow5\sqrt{x}-x=2\sqrt{x}\)

\(\Leftrightarrow x-5\sqrt{x}+2\sqrt{x}=0\)

\(\Leftrightarrow x-3\sqrt{x}=0\)

\(\Leftrightarrow\sqrt{x}\left(\sqrt{x}-3\right)=0\)

hay x=9

a)

\(P=\dfrac{\left(\sqrt{x}-1\right)\left(3\sqrt{x}+1\right)-\left(3\sqrt{x}-4\right)+5\sqrt{x}}{\left(3\sqrt{x}+1\right)\left(3\sqrt{x}-1\right)}.\dfrac{3\sqrt{x}+1}{3}\)

\(P=\dfrac{3x-2\sqrt{x}-1-3\sqrt{x}+4+5\sqrt{x}}{\left(3\sqrt{x}+1\right)\left(3\sqrt{x}-1\right)}.\dfrac{3\sqrt{x}+1}{3}\)

\(P=\dfrac{3\left(x+1\right)}{\left(3\sqrt{x}+1\right)\left(3\sqrt{x}-1\right)}.\dfrac{3\sqrt{x}+1}{3}\)

\(P=\dfrac{x+1}{3\sqrt{x}-1}\)

b) Từ phương trình suy ra \(\left[{}\begin{matrix}x=1\\x=\dfrac{1}{9}\end{matrix}\right.\)

Vói x=1 

\(P=\dfrac{1}{3\sqrt{1}-1}=\dfrac{1}{2}\)

Với x= 1/9

\(P=\dfrac{\dfrac{1}{9}}{3\sqrt{\dfrac{1}{9}}-1}\) không có nghiệm

a: ĐKXĐ: \(x\in R\)

\(\sqrt{x^2-4x+4}=7\)

=>\(\sqrt{\left(x-2\right)^2}=7\)

=>|x-2|=7

=>\(\left[{}\begin{matrix}x-2=7\\x-2=-7\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=9\\x=-5\end{matrix}\right.\)

b: ĐKXĐ: x>=-3

\(\sqrt{4x+12}-3\sqrt{x+3}+\dfrac{4}{3}\cdot\sqrt{9x+27}=6\)

=>\(2\sqrt{x+3}-3\sqrt{x+3}+\dfrac{4}{3}\cdot3\sqrt{x+3}=6\)

=>\(3\sqrt{x+3}=6\)

=>\(\sqrt{x+3}=2\)

=>x+3=4

=>x=1(nhận)

a) ⇔ |2x+3| = 8
⇒ \(\left[{}\begin{matrix}2x+3=8\\2x+3=-8\end{matrix}\right.\) ⇔ \(\left[{}\begin{matrix}2x=5\\2x=-11\end{matrix}\right.\) ⇔ \(\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=-\dfrac{11}{2}\end{matrix}\right.\)

Vậy...

b) ĐKXĐ: \(x\ge0\)

\(\Leftrightarrow3\sqrt{x}-7\sqrt{x}+6\sqrt{x}=8\)

\(\Leftrightarrow2\sqrt{x}=8\)

\(\Leftrightarrow\sqrt{x}=4\)

\(\Leftrightarrow x=16\) (Vì \(x\ge0\) )

Vậy x = 16

c) ĐKXĐ: \(x\ge1\)

\(\Leftrightarrow\sqrt{9\left(x-1\right)}=12\)

\(\Leftrightarrow3\sqrt{x-1}=12\)

\(\Leftrightarrow\sqrt{x-1}=4\)

\(\Leftrightarrow x-1=16\)

\(\Leftrightarrow x=17\)(TM)

Vậy x = 17

`a)sqrt{9x^2}=6`

`<=>|3x|=6`

`<=>|x|=2`

`<=>x=+-2`

`b)sqrt{(x-2)^2}=5`

`<=>|x-2|=5`

`**x-2=5`

`<=>x=7`

`**x-2=-5`

`<=>x=-3`

`c)sqrt{x^2-6x+9}=3`

`<=>\sqrt{(x-3)^2}=3`

`<=>|x-3|=3`

`**x-3=3`

`<=>x=6`

`**x-3=-3`

`<=>x=0`

`d)sqrt{x^2+4x+4}-2x=3`

`<=>sqrt{(x+2)^2}=3+2x`

`<=>|x+2|=2x+3(x>=-3/2)`

`**x+2=2x+3`

`<=>x=-1(tm)`

`**x+2=-2x-3`

`<=>3x=-5`

`<=>x=-5/3(l)`

Sử dụng công thức:`sqrtA^2=|A|`

ĐKXĐ : \(x\in R\)

a, \(\sqrt{9x^2}=\left|3x\right|=6\)

\(\Leftrightarrow\left[{}\begin{matrix}3x=6\\3x=-6\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-2\end{matrix}\right.\)

Vậy ..

b, \(\sqrt{\left(x-2\right)^2}=\left|x-2\right|=5\)

\(\Leftrightarrow\left[{}\begin{matrix}x-2=5\\x-2=-5\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=7\\x=-3\end{matrix}\right.\)

Vậy ...

c, \(\sqrt{x^2-6x+9}=\sqrt{\left(x-3\right)^2}=\left|x-3\right|=3\)

\(\Leftrightarrow\left[{}\begin{matrix}x-3=3\\x-3=-3\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=6\\x=0\end{matrix}\right.\)

Vậy ..

d, \(\sqrt{x^2+4x+4}-2x=\sqrt{\left(x+2\right)^2}-2x=\left|x+2\right|-2x=3\)

\(\Leftrightarrow\left|x+2\right|=2x+3\)

\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x+2=2x+3\\x+2=-2x-3\end{matrix}\right.\\2x+3\ge0\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x\ge-\dfrac{3}{2}\\\left[{}\begin{matrix}x=-1\left(TM\right)\\x=-\dfrac{5}{3}\left(L\right)\end{matrix}\right.\end{matrix}\right.\)

Vậy ..

`a)sqrt{x^2-2x+1}=2`

`<=>sqrt{(x-1)^2}=2`

`<=>|x-1|=2`

`**x-1=2<=>x=3`

`**x-1=-1<=>x=-1`.

Vậy `S={3,-1}`

`b)sqrt{x^2-1}=x`

Điều kiện:\(\begin{cases}x^2-1 \ge 0\\x \ge 0\\\end{cases}\)

`<=>` \(\begin{cases}x^2 \ge 1\\x \ge 0\\\end{cases}\)

`<=>x>=1`

`pt<=>x^2-1=x^2`

`<=>-1=0` vô lý

Vậy pt vô nghiệm

`c)sqrt{4x-20}+3sqrt{(x-5)/9}-1/3sqrt{9x-45}=4(x>=5)`

`pt<=>sqrt{4(x-5)}+sqrt{9*(x-5)/9}-sqrt{(9x-45)*1/9}=4`

`<=>2sqrt{x-5}+sqrt{x-5}-sqrt{x-5}=4`

`<=>2sqrt{x-5}=4`

`<=>sqrt{x-5}=2`

`<=>x-5=4`

`<=>x=9(tmđk)`

Vậy `S={9}.`

`d)x-5sqrt{x-2}=-2(x>=2)`

`<=>x-2-5sqrt{x-2}+4=0`

Đặt `a=sqrt{x-2}`

`pt<=>a^2-5a+4=0`

`<=>a_1=1,a_2=4`

`<=>sqrt{x-2}=1,sqrt{x-2}=4`

`<=>x_1=3,x_2=18`,

`e)2x-3sqrt{2x-1}-5=0`

`<=>2x-1-3sqrt{2x-1}-4=0`

Đặt `a=sqrt{2x-1}(a>=0)`

`pt<=>a^2-3a-4=0`

`a-b+c=0`

`<=>a_1=-1(l),a_2=4(tm)`

`<=>sqrt{2x-1}=4`

`<=>2x-1=16`

`<=>x=17/2(tm)`

Vậy `S={17/2}`

d.

ĐKXĐ: $x\geq 2$. Đặt $\sqrt{x-2}=a(a\geq 0)$ thì pt trở thành:

$a^2+2-5a=-2$

$\Leftrightarrow a^2-5a+4=0$

$\Leftrightarrow (a-1)(a-4)=0$

$\Rightarrow a=1$ hoặc $a=4$

$\Leftrightarrow \sqrt{x-2}=1$ hoặc $\sqrt{x-2}=4$

$\Leftrightarrow x=3$ hoặc $x=18$ (đều thỏa mãn)

e. ĐKXĐ: $x\geq \frac{1}{2}$

Đặt $\sqrt{2x-1}=a(a\geq 0)$ thì pt trở thành:

$a^2+1-3a-5=0$

$\Leftrightarrow a^2-3a-4=0$

$\Leftrightarrow (a+1)(a-4)=0$

Vì $a\geq 0$ nên $a=4$

$\Leftrightarrow \sqrt{2x-1}=4$

$\Leftrightarrow x=\frac{17}{2}$

a.

$\sqrt{x^2-2x+1}=2$

$\Leftrightarrow \sqrt{(x-1)^2}=2$

$\Leftrightarrow |x-1|=2$

$\Rightarrow x-1=\pm 2$

$\Leftrightarrow x=3$ hoặc $x=-1$ (đều thỏa mãn)

b. ĐKXĐ: $x\geq 1$ hoặc $x\leq -1$

PT \(\Rightarrow \left\{\begin{matrix} x\geq 0\\ x^2-1=x^2\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq 0\\ 1=0\end{matrix}\right.\) (vô lý)

Vậy pt vô nghiệm

c. ĐKXĐ: $x\geq 5$

PT $\Leftrightarrow \sqrt{4(x-5)}+3\sqrt{\frac{x-5}{9}}-\frac{1}{3}\sqrt{9(x-5)}=4$

$\Leftrightarrow 2\sqrt{x-5}+\sqrt{x-5}-\sqrt{x-5}=4$

$\Leftrightarrow 2\sqrt{x-5}=4$

$\Leftrightarrow \sqrt{x-5}=2$

$\Leftrightarrow x=2^2+5=9$ (thỏa mãn)

Tìm x để căn có nghĩa ak mn giúp e với ak

\(a,ĐK:\dfrac{3}{x+7}\ge0\Leftrightarrow x+7>0\left(3>0;x+7\ne0\right)\Leftrightarrow x>-7\\ b,ĐK:\dfrac{-2}{5-x}\ge0\Leftrightarrow5-x< 0\left(2-< 0;5-x\ne0\right)\Leftrightarrow x>5\\ c,ĐK:x^2-7x+10\ge0\Leftrightarrow\left(x-5\right)\left(x-2\right)\ge0\\ \Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x-5\ge0\\x-2\ge0\end{matrix}\right.\\\left\{{}\begin{matrix}x-5\le0\\x-2\le0\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x\ge5\\x\le2\end{matrix}\right.\)

\(d,ĐK:x^2-8x+10\ge0\Leftrightarrow\left(x-4-\sqrt{6}\right)\left(x-4+\sqrt{6}\right)\ge0\\ \Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x-4-\sqrt{6}\ge0\\x-4+\sqrt{6}\ge0\end{matrix}\right.\\\left\{{}\begin{matrix}x-4-\sqrt{6}\le0\\x-4+\sqrt{6}\le0\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x\ge4+\sqrt{6}\\x\ge4-\sqrt{6}\end{matrix}\right.\\\left\{{}\begin{matrix}x\le4+\sqrt{6}\\x\le4-\sqrt{6}\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x\ge4+\sqrt{6}\\x\le4-\sqrt{6}\end{matrix}\right.\)

\(e,ĐK:9x^2+1\ge0\Leftrightarrow x\in R\left(9x^2+1\ge1>0\right)\)

a) \(ĐK:x+7>0\Leftrightarrow x>-7\)

b) \(ĐK:5-x< 0\Leftrightarrow x>5\)

c) \(ĐK:x^2-7x+10\ge0\)

\(\Leftrightarrow\left(x-2\right)\left(x-5\right)\ge0\)

\(\Leftrightarrow\left[{}\begin{matrix}x\ge5\\x\le2\end{matrix}\right.\)

d) \(ĐK:x^2-8x+10\ge0\)

\(\Leftrightarrow\left(x-4-\sqrt{6}\right)\left(x-4+\sqrt{6}\right)\ge0\)

\(\Leftrightarrow\left[{}\begin{matrix}x\ge4+\sqrt{6}\\x\le4-\sqrt{6}\end{matrix}\right.\)

e) Do \(9x^2+1\ge1>0\)

Nên biểu thức được xác định với mọi x

\(1,\left[{}\begin{matrix}\left\{{}\begin{matrix}x-6\ge0\\x-2>0\end{matrix}\right.\\\left\{{}\begin{matrix}x-6\le0\\x-2< 0\end{matrix}\right.\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x\ge6\\x< 2\end{matrix}\right.\\ 2,4-9x^2\ge0\\ \Leftrightarrow-\dfrac{2}{3}\le x\le\dfrac{2}{3}\)

\(3,x^2-8x-9\ge0\\ \Leftrightarrow\left(x+1\right)\left(x-9\right)\ge0\Leftrightarrow\left[{}\begin{matrix}x\ge9\\x\le-1\end{matrix}\right.\)

1: ĐKXĐ: (x-6)/(x-2)>=0

=>x>=6 hoặc x<2

2: ĐKXĐ: 4-9x^2>=0

=>9x^2<=4

=>x^2<=4/9

=>-2/3<=x<=2/3

3: ĐKXĐ: x^2-8x-9>=0

=>(x-9)(x+1)>=0

=>x>=9 hoặc x<=-1

\(a,\Leftrightarrow x-1=4\Leftrightarrow x=5\\ b,\Leftrightarrow\left\{{}\begin{matrix}x\ge\dfrac{3}{4}\\3x+1=4x-3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ge\dfrac{3}{4}\\x=4\left(tm\right)\end{matrix}\right.\Leftrightarrow x=4\\ c,ĐK:x\ge-5\\ PT\Leftrightarrow2\sqrt{x+5}-3\sqrt{x+5}+4\sqrt{x+5}=6\\ \Leftrightarrow3\sqrt{x+5}=6\\ \Leftrightarrow\sqrt{x+5}=3\\ \Leftrightarrow x+5=9\\ \Leftrightarrow x=4\left(tm\right)\)

\(d,\Leftrightarrow\sqrt{\left(x-2\right)^2}=\sqrt{\left(\sqrt{5}+1\right)^2}\\ \Leftrightarrow\left|x-2\right|=\sqrt{5}+1\\ \Leftrightarrow\left[{}\begin{matrix}x-2=\sqrt{5}+1\\2-x=\sqrt{5}+1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\sqrt{5}+3\\x=1-\sqrt{5}\end{matrix}\right.\)