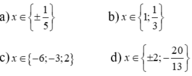\(x^4+2x^3-2x^2+2x-3=0\\ \Leftrightarrow x^4+3x^3-x^3-3x^2+x^2+3x-x-3=0\\ \Leftrightarrow x^3\left(x+3\right)-x^2\left(x+3\right)+x\left(x+3\right)-\left(x+3\right)=0\\ \Leftrightarrow\left(x+3\right)\left(x^3-x^2+x-1\right)=0\\ \Leftrightarrow\left(x+3\right)\left[x^2\left(x-1\right)+\left(x-1\right)\right]=0\\ \Leftrightarrow\left(x+3\right)\left(x-1\right)\left(x^2+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x+3=0\\x-1=0\\x^2+1=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-3\\x=1\end{matrix}\right.\left(\text{vì }x^2+1\ge1>0\right)\)

Vậy ...

\(\left(x-1\right)\left(x^2+5x-2\right)-x^3+1=0\\ \Leftrightarrow\left(x-1\right)\left(x^2+5x-2\right)-\left(x^3-1\right)=0\\ \Leftrightarrow\left(x-1\right)\left(x^2+5x-2\right)-\left(x-1\right)\left(x^2+x+1\right)=0\\ \Leftrightarrow\left(x-1\right)\left[\left(x^2+5x-2\right)-\left(x^2+x+1\right)\right]=0\\ \Leftrightarrow\left(x-1\right)\left(4x-3\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-1=0\\4x-3=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=1\\x=\dfrac{3}{4}\end{matrix}\right.\)

Vậy ...

\(x^2+\left(x+2\right)\left(11x-7\right)=4\\ \Leftrightarrow x^2-4+\left(x+2\right)\left(11x-7\right)=0\\ \Leftrightarrow\left(x+2\right)\left(x-2\right)+\left(11x-7\right)=0\\ \Leftrightarrow\left(x+2\right)\left(x-2+11x-7\right)=0\\ \Leftrightarrow\left(x+2\right)\left(12x-9\right)=0\\ \Leftrightarrow3\left(x+2\right)\left(4x-3\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x+2=0\\4x-3=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-2\\x=\dfrac{3}{4}\end{matrix}\right.\)

Vậy ...

Q=(x1+x2)^2-2x1x2+6x1x2

=(-5)^2+4*(-4)

=25-16=9

Áp dụng Viét có: `{(x_1+x_2=-b/a=-5),(x_1.x_2=c/a=-4):}`

Ta có: `Q=(x_1+x_2)^2+4x_1.x_2`

`<=>Q=(-5)^2+4.(-4)`

`<=>Q=9`

\(\Leftrightarrow x^3-3x^2+2-\left(3x^2-2x-1\right)m=0\)

\(\Leftrightarrow\left(x-1\right)\left(x^2-2x-2\right)-\left(x-1\right)\left(3mx+m\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left(x^2-\left(3m+2\right)x-m-2\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x^2-\left(3m+2\right)x-m-2=0\left(1\right)\end{matrix}\right.\)

(1) luôn có 2 nghiệm pb. Để pt có 3 nghiệm pb \(\Rightarrow1-\left(3m+2\right)-m-2\ne0\Rightarrow m\ne-\dfrac{3}{4}\)

TH1: \(x_3=1\) và \(x_1;x_2\) là nghiệm của (1)

\(\Rightarrow3m+2=2\Rightarrow m=0\) (thỏa mãn)

TH2: \(x_1=1\) và \(x_2;x_3\) là nghiệm của (1)

Kết hợp hệ thức Viet ta được: \(\left\{{}\begin{matrix}x_2=2x_3-1\\x_2+x_3=3m+2\end{matrix}\right.\)

\(\Rightarrow\left\{{}\begin{matrix}x_2=2x_3-1\\x_3=m+1\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x_2=2m+1\\x_3=m+1\end{matrix}\right.\)

Thế vào \(x_2x_3=-m-2\)

\(\Rightarrow\left(2m+1\right)\left(m+1\right)=-m-2\)

\(\Rightarrow2m^2+4m+3=0\) (vô nghiệm)

Vậy \(m=0\)

Ta có: \(\dfrac{5}{-x^2+5x-6}+\dfrac{x+3}{2-x}=0\)

\(\Leftrightarrow\dfrac{-5}{\left(x-2\right)\left(x-3\right)}-\dfrac{x^2-9}{\left(x-2\right)\left(x-3\right)}=0\)

\(\Leftrightarrow-5-x^2+9=0\)

\(\Leftrightarrow x=-2\)

a: (2x+1)(3-x)(4-2x)=0

=>(2x+1)(x-3)(x-2)=0

hay \(x\in\left\{-\dfrac{1}{2};3;2\right\}\)

b: 2x(x-3)+5(x-3)=0

=>(x-3)(2x+5)=0

=>x=3 hoặc x=-5/2

c: =>(x-2)(x+2)+(x-2)(2x-3)=0

=>(x-2)(x+2+2x-3)=0

=>(x-2)(3x-1)=0

=>x=2 hoặc x=1/3

d: =>(x-2)(x-3)=0

=>x=2 hoặc x=3

e: =>(2x+5+x+2)(2x+5-x-2)=0

=>(3x+7)(x+3)=0

=>x=-7/3 hoặc x=-3

f: \(\Leftrightarrow2x^3+5x^2-3x=0\)

\(\Leftrightarrow x\left(2x^2+5x-3\right)=0\)

\(\Leftrightarrow x\left(x+3\right)\left(2x-1\right)=0\)

hay \(x\in\left\{0;-3;\dfrac{1}{2}\right\}\)

Viết rõ đề bài ra bạn.

d: Ta có: \(\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)=24\)

\(\Leftrightarrow\left(x^2+5x+4\right)\left(x^2+5x+6\right)-24=0\)

\(\Leftrightarrow x\left(x+5\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-5\end{matrix}\right.\)

Ta có: \(2x^3-x^2+5x+3\)

\(=2x^3+x^2-2x^2-x+6x+3\)

\(=x^2\left(2x+1\right)-x\left(2x+1\right)+3\left(2x+1\right)\)

\(=\left(2x+1\right)\left(x^2-x+3\right)\)

`2x^3-x^2+5x+3`

`=2x^3-2x^2+6x+x^2-x+3`

`=2x(x^2-x+3)+(x^2-x+3)`

`=(2x+1)(x^2-x+3)`