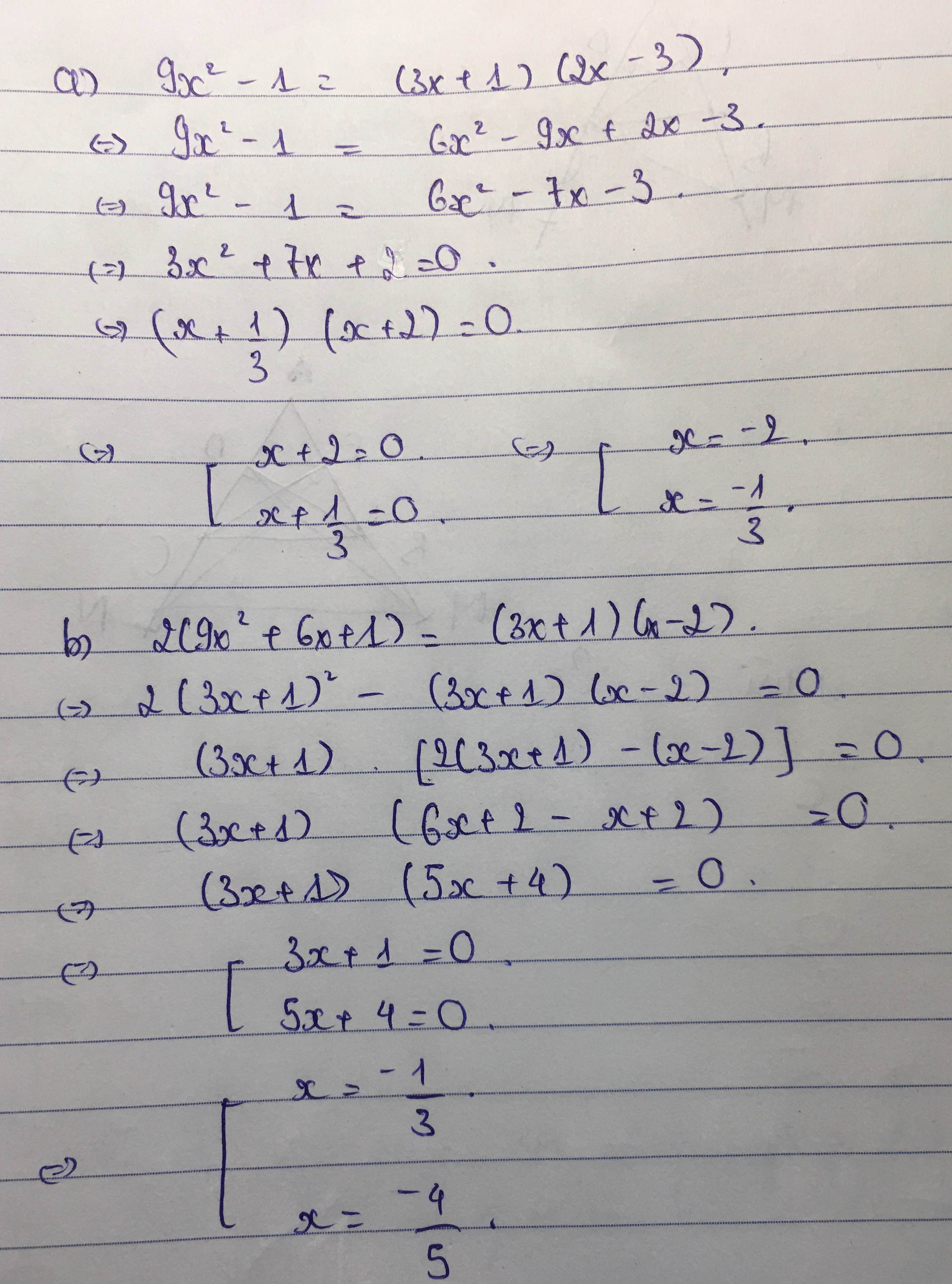`a)\sqrt{3x}-5\sqrt{12x}+7\sqrt{27x}=12`     `ĐK: x >= 0`

`<=>\sqrt{3x}-10\sqrt{3x}+21\sqrt{3x}=12`

`<=>12\sqrt{3x}=12`

`<=>\sqrt{3x}=1`

`<=>3x=1<=>x=1/3` (t/m)

`b)5\sqrt{9x+9}-2\sqrt{4x+4}+\sqrt{x+1}=36`   `ĐK: x >= -1`

`<=>15\sqrt{x+1}-4\sqrt{x+1}+\sqrt{x+1}=36`

`<=>12\sqrt{x+1}=36`

`<=>\sqrt{x+1}=3`

`<=>x+1=9`

`<=>x=8` (t/m)

Ta có hệ \(\hept{\begin{cases}x^2+y^2-3x+4y=1\\3x^2-2y^2-9x-8y=3\end{cases}\Leftrightarrow\hept{\begin{cases}3x^2+3y^2-9x+12y=3\left(1\right)\\3x^2-2y^2-9x-8y=3\left(2\right)\end{cases}}}\)

Lấy (1)-(2) ta có \(5y^2+20y=0\Leftrightarrow\orbr{\begin{cases}y=0\\y=-4\end{cases}}\)

Với \(y=0\Rightarrow x^2-3x-1=0\Leftrightarrow\orbr{\begin{cases}x=\frac{3+\sqrt{13}}{2}\\x=\frac{3-\sqrt{13}}{2}\end{cases}}\)

Với \(y=-4\Rightarrow x^2-3x-1=0\Leftrightarrow\orbr{\begin{cases}x=\frac{3+\sqrt{13}}{2}\\x=\frac{3-\sqrt{13}}{2}\end{cases}}\)

Vậy hệ có 4 nghiệm \(\left(x;y\right)=\left(0;\frac{3+\sqrt{13}}{2}\right);\left(0;\frac{3-\sqrt{13}}{2}\right);\left(-4;\frac{3+\sqrt{13}}{2}\right);\left(-4;\frac{3-\sqrt{13}}{2}\right)\)

a/ 3x(12x-4)-9x(4x-3)

=36x²-12x-36x²+27x

=(36x²-36x²)-12x+27x

=15x

b/ x(5-2x)+2x(x-1)

=5x-2x²+2x²-2x

=(5x-2x)-(-2x²+2x²)

=3x

c/ 5x(12x+7)-3x(20x-5)

=60x²+35x-60x²+15x

=(60x²-60x²)+(35x+15x)\

=50x

d/ 3x(2x-7)+2x(5-3x)

=6x²-21x+10x-6x²

=(6x²-6x²)+(10x-21x)

=-11x

e/ đề sai hay sao ý ra số to lắm @@

a,\(\sqrt{\left(3x-1\right)^2}=5=>|3x-1|=5=>\left[{}\begin{matrix}3x-1=5\\3x-1=-5\end{matrix}\right.\)

\(=>\left[{}\begin{matrix}x=2\\x=-\dfrac{4}{3}\end{matrix}\right.\)

b, \(\sqrt{4x^2-4x+1}=3=\sqrt{\left(2x-1\right)^2}=3=>\left[{}\begin{matrix}2x-1=3\\2x-1=-3\end{matrix}\right.\)

\(=>\left[{}\begin{matrix}x=2\\x=-1\end{matrix}\right.\)

c, \(\sqrt{x^2-6x+9}+3x=4=>|x-3|=4-3x\)

TH1: \(|x-3|=x-3< =>x\ge3=>x-3=4-3x=>x=1,75\left(ktm\right)\)

TH2 \(|x-3|=3-x< =>x< 3=>3-x=4-3x=>x=0,5\left(tm\right)\)

Vậy x=0,5...

d, đk \(x\ge-1\)

=>pt đã cho \(< =>9\sqrt{x+1}-6\sqrt{x+1}+4\sqrt{x+1}=12\)

\(=>7\sqrt{x+1}=12=>x+1=\dfrac{144}{49}=>x=\dfrac{95}{49}\left(tm\right)\)

a) Ta có: \(\sqrt{\left(3x-1\right)^2}=5\)

\(\Leftrightarrow\left|3x-1\right|=5\)

\(\Leftrightarrow\left[{}\begin{matrix}3x-1=5\\3x-1=-5\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}3x=6\\3x=-4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-\dfrac{4}{3}\end{matrix}\right.\)

b) Ta có: \(\sqrt{4x^2-4x+1}=3\)

\(\Leftrightarrow\left|2x-1\right|=3\)

\(\Leftrightarrow\left[{}\begin{matrix}2x-1=3\\2x-1=-3\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x=4\\2x=-2\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-1\end{matrix}\right.\)

c) Ta có: \(\sqrt{x^2-6x+9}+3x=4\)

\(\Leftrightarrow\left|x-3\right|=4-3x\)

\(\Leftrightarrow\left[{}\begin{matrix}x-3=4-23x\left(x\ge3\right)\\x-3=23x-4\left(x< 3\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x+23x=4+3\\x-23x=4+3\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{7}{24}\left(loại\right)\\x=\dfrac{-4}{22}=\dfrac{-2}{11}\left(loại\right)\end{matrix}\right.\)

a: =>(3x+1)(3x-1)-(3x+1)(2x-3)=0

=>(3x+1)(3x-1-2x+3)=0

=>(3x+1)(x+2)=0

=>x=-1/3 hoặc x=-2

b: =>(3x+1)(6x+2)-(3x+1)(x-2)=0

=>(3x+1)(6x+2-x+2)=0

=>(3x+1)(5x+4)=0

=>x=-1/3 hoặc x=-4/5

Lời giải:

\(\text{HPT}\Leftrightarrow \left\{\begin{matrix} 7(2x^3+3x^2y)=35\\ 5(y^3+6xy^2)=35\end{matrix}\right.\Rightarrow 14x^3+21x^2y-5y^3-30xy^2=0(1)\)

Nhận thấy $x,y\neq 0$ nên đặt \(x=ty(t\neq 0)\). Thay vào $(1)$ ta được:

\(14t^3y^3+21t^2y^3-5y^3-30ty^3=0\)

\(\Leftrightarrow 14t^3+21t^2-30t-5=0\Leftrightarrow (t-1)(14t^2+35t+5)=0\)

Nếu \(t=1\Rightarrow x=y\rightarrow 7y^3=7\Rightarrow y=1\rightarrow x=1\)

Nếu \(14t^2+35t+5=0\Rightarrow \left[ \begin{array}{ll}t=\frac{-35+3\sqrt{105}}{28} \\ \\ t=\frac{-35-3\sqrt{105}}{28}\end{array} \right.\)

Ta có \(y^3+6xy^2=y^3+6ty^3=7\Rightarrow y^3=\frac{7}{6t+1}\)

Thay vào ta tìm được \(\left[ \begin{array}{ll}y=\frac{7+\sqrt{105}}{4} \rightarrow x=\frac{5-\sqrt{105}}{8} \\ \\ y=\frac{7-\sqrt{105}}{4}\rightarrow x=\frac{5+\sqrt{105}}{8}\end{array} \right.\)

Ta có cặp nghiệm \((x,y)=(1,1),\left ( \frac{5+\sqrt{105}}{8},\frac{7-\sqrt{105}}{4} \right ),\left ( \frac{5-\sqrt{105}}{8},\frac{7+\sqrt{105}}{4} \right )\)