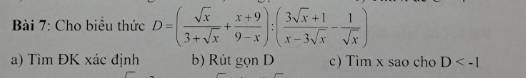a: ĐKXĐ: x>0; x<>9
b: \(D=\dfrac{\sqrt{x}\left(3-\sqrt{x}\right)+x+9}{9-x}:\dfrac{3\sqrt{x}+1-\sqrt{x}+3}{\sqrt{x}\left(\sqrt{x}-3\right)}\)
\(=\dfrac{3\sqrt{x}+9}{-\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\cdot\dfrac{\sqrt{x}\left(\sqrt{x}-3\right)}{2\sqrt{x}+4}\)
\(=\dfrac{-3\sqrt{x}}{2\sqrt{x}+4}\)
c: D<-1
=>D+1<0
=>\(\dfrac{-3\sqrt{x}+2\sqrt{x}+4}{2\sqrt{x}+4}< 0\)
=>\(-\sqrt{x}+4< 0\)
=>x>16
a) ĐKXĐ: \(x>0;x\ne9\)
b) \(D=\left(\dfrac{\sqrt{x}}{3+\sqrt{x}}+\dfrac{x+9}{9-x}\right):\left(\dfrac{3\sqrt{x}+1}{x-3\sqrt{x}}-\dfrac{1}{\sqrt{x}}\right)\)
\(=\left[\dfrac{\sqrt{x}\left(\sqrt{x}-3\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}-\dfrac{x+9}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\right]:\left[\dfrac{3\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-3\right)}-\dfrac{\sqrt{x}-3}{\sqrt{x}\left(\sqrt{x}-3\right)}\right]\)
\(=\left[\dfrac{x-3\sqrt{x}-x-9}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\right]:\left[\dfrac{3\sqrt{x}+1-\sqrt{x}+3}{\sqrt{x}\left(\sqrt{x}-3\right)}\right]\)
\(=\left[\dfrac{-3\sqrt{x}-9}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\right]:\dfrac{2\sqrt{x}+4}{\sqrt{x}\left(\sqrt{x}-3\right)}\)
\(=\left[\dfrac{-3\left(\sqrt{x}+3\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\right]:\dfrac{2\sqrt{x}+4}{\sqrt{x}\left(\sqrt{x}-3\right)}\)
\(=\dfrac{-3}{\sqrt{x}-3}\cdot\dfrac{\sqrt{x}\left(\sqrt{x}-3\right)}{2\sqrt{x}+4}\)
\(=\dfrac{-3\sqrt{x}}{2\sqrt{x}+4}\)
c) Với \(x>0;x\ne9\) thì \(D< -1\)
\(\Leftrightarrow\dfrac{-3\sqrt{x}}{2\sqrt{x}+4}< -1\)
\(\Leftrightarrow\dfrac{-3\sqrt{x}}{2\sqrt{x}+4}+1< 0\)
\(\Leftrightarrow\dfrac{-3\sqrt{x}}{2\sqrt{x}+4}+\dfrac{2\sqrt{x}+4}{2\sqrt{x}+4}< 0\)
\(\Leftrightarrow\dfrac{-\sqrt{x}+4}{2\sqrt{x}+4}< 0\)
\(\Rightarrow-\sqrt{x}+4< 0\left(vì2\sqrt{x}+4>0\forall x\right)\)
\(\Rightarrow-\sqrt{x}< -4\)
\(\Rightarrow\sqrt{x}>4\)
\(\Rightarrow x>16\)
Kết hợp với điều kiện, ta được: \(x>16\)
#Ayumu