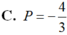\(\dfrac{3\pi}{2}< x< 2\pi\Rightarrow sinx< 0\Rightarrow sinx=-\sqrt{1-cos^2x}=-\dfrac{2\sqrt{2}}{3}\)
\(tanx=\dfrac{sinx}{cosx}=-2\sqrt{2}\)
\(sin2x=2sinx.cosx=-\dfrac{4\sqrt{2}}{9}\)
\(cos2x=2cos^2x-1=-\dfrac{7}{9}\)
\(\Rightarrow sin\left(2x-\dfrac{\pi}{4}\right)=sin2x.cos\left(\dfrac{\pi}{4}\right)-cos2x.sin\left(\dfrac{\pi}{4}\right)=-\dfrac{4\sqrt{2}}{9}.\dfrac{\sqrt{2}}{2}-\left(-\dfrac{7}{9}\right).\dfrac{\sqrt{2}}{2}=...\)
\(sin4x=2sin2x.cos2x=2.\left(-\dfrac{4\sqrt{2}}{9}\right).\left(-\dfrac{7}{9}\right)=...\)
Đúng 1
Bình luận (0)
