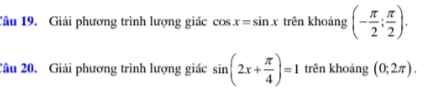a) \(2cosx.cos2x.cos3x-7=7cos2x\)
\(\Leftrightarrow\left(cos2x+cos4x\right).cos2x=7\left(cos2x+1\right)\)
\(\Leftrightarrow\left(cos2x+2cos^22x-1\right)cos2x=7\left(cos2x+1\right)\)
\(\Leftrightarrow\left(cos2x+1\right)\left(2cos2x-1\right)cos2x=7\left(1+cos2x\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x+1=0\\2cos^22x-cos2x=7\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}cos2x+1=0\\cos2x=\dfrac{1+\sqrt{57}}{4}\left(vn\right)\\cos2x=\dfrac{1-\sqrt{57}}{4}\left(vn\right)\end{matrix}\right.\)\(\Rightarrow2x=\pi+k2\pi,k\in Z\)
\(\Leftrightarrow x=\dfrac{\pi}{2}+k\pi\) ( k nguyên)
Vậy...
c) Pt\(\Leftrightarrow2sin^3x+2sin^2x-1+cosx=0\)
\(\Leftrightarrow2sin^2x\left(sinx+1\right)+\left(cosx-1\right)=0\)
\(\Leftrightarrow\left(1-cosx\right)\left(1+cosx\right)\left(2sinx+2\right)+\left(cosx-1\right)=0\)
\(\Leftrightarrow\left(1-cosx\right)\left(2sinx+2sinx.cosx+2cosx+1\right)=0\)
\(\Leftrightarrow\left(1-cosx\right)\left[2\left(sinx+cosx\right)+\left(sin^2x+2sinx.cosx+cos^2x\right)\right]=0\)
\(\Leftrightarrow\left(1-cosx\right)\left(sinx+cosx\right)\left(2+sinx+cosx\right)=0\) (I)
Có \(sinx+cosx=\sqrt{2}sin\left(x+\dfrac{\pi}{4}\right)\ge-\sqrt{2}\)
\(\Rightarrow2+sinx+cosx\ge2-\sqrt{2}>0\)
Từ (I)\(\Rightarrow\left[{}\begin{matrix}1-cosx=0\\sinx+cosx=0\end{matrix}\right.\)\(\Rightarrow\left[{}\begin{matrix}cosx=1\\tanx=-1\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=k2\pi\\x=\dfrac{-\pi}{4}+k\pi\end{matrix}\right.\)\(\left(k\in Z\right)\)
Vậy...
e) Pt \(\Leftrightarrow8sinx.cosx-3\left(1-2sin^2x\right)=12sinx-3\)
\(\Leftrightarrow8sinx.cosx+6sin^2x=12sinx\)
\(\Leftrightarrow2sinx\left(4cosx+3sinx-6\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=0\\4cosx+3sinx=6\left(vn\right)\end{matrix}\right.\)(vô nghiệm vì 42+32<62)\(\Rightarrow x=k\pi\) \(\left(k\in Z\right)\)
Vậy...